| Date | May 2014 | Marks available | 4 | Reference code | 14M.2.sl.TZ1.4 |
| Level | SL only | Paper | 2 | Time zone | TZ1 |
| Command term | Find | Question number | 4 | Adapted from | N/A |
Question
ABC is a triangular field on horizontal ground. The lengths of AB and AC are 70 m and 50 m respectively. The size of angle BCA is 78°.
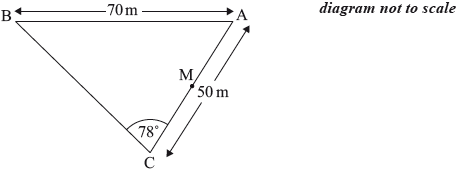
Find the size of angle ABC.
Find the area of the triangular field.
M is the midpoint of AC.
Find the length of BM.
A vertical mobile phone mast, TB, is built next to the field with its base at B. The angle of elevation of T from M is 63.4∘. N is the midpoint of the mast.

Calculate the angle of elevation of N from M.
Markscheme
70sin78=50sinAˆBC (M1)(A1)
Note: Award (M1) for substituted sine rule, (A1) for correct substitution.
AˆBC=44.3∘ (44.3209...) (A1)(G3)
Note: If radians are used the answer is 0.375918..., award at most (M1)(A1)(A0).
[3 marks]
area ΔABC=12×70×50×sin(57.6790…) (A1)(M1)(A1)(ft)
Notes: Award (A1)(ft) for their 57.6790… seen, (M1) for substituted area formula, (A1)(ft) for correct substitution.
Follow through from part (a).
=1480 m2 (1478.86…) (A1)(ft)(G3)
Notes: The answer is 1480 m2, units are required. 1479.20… if 3 sf used.
If radians are used the answer is 1554.11…m2, award (A1)(ft)(M1)(A1)(ft)(A1)(ft)(G3).
[4 marks]
BM2=702+252−2×70×25×cos(57.6790…) (M1)(A1)(ft)
Notes: Award (M1) for substituted cosine rule, (A1)(ft) for correct substitution. Follow through from their angle in part (b).
BM=60.4 (m) (60.4457…) (A1)(ft)(G2)
Notes: If the 3 sf answer is used the answer is 60.5 (m).
If radians are used the answer is 62.5757… (m), award (M1)(A1)(ft)(A1)(ft)(G2).
[3 marks]
tan63.4∘=TB60.4457… (M1)
Note: Award (M1) for their correctly substituted trig equation.
TB=120.707… (A1)(ft)
Notes: Follow through from part (c). If 3 sf answers are used throughout, TB=120.815…
If TB=120.707… is seen without working, award (A2).
tanNˆMB=(120.707…2)60.4457… (A1)(ft)(M1)
Notes: Award (A1)(ft) for their TB divided by 2 seen, (M1) for their correctly substituted trig equation.
Follow through from part (c) and within part (d).
NˆMB=45.0∘ (44.9563…) (A1)(ft)(G3)
Notes: If 3 sf are used throughout, answer is 45∘.
If radians are used the answer is 0.308958…, and if full working is shown, award at most (M1)(A1)(ft)(A1)(ft)(M1)(A0).
If no working is shown for radians answer, award (G2).
OR
tanNˆMB=NBBM (M1)
tan63.4∘=2×NBBM (A1)(M1)
Note: Award (A1) for 2×NB seen.
tanNˆMB=12tan63.4∘ (M1)
NˆMB=45.0∘ (44.9563…) (A1)(G3)
Notes: If radians are used the answer is 0.308958…, and if full working is shown, award at most (M1)(A1)(M1)(M1)(A0). If no working is shown for radians answer, award (G2).
[5 marks]

