| Date | May 2015 | Marks available | 3 | Reference code | 15M.1.sl.TZ2.12 |
| Level | SL only | Paper | 1 | Time zone | TZ2 |
| Command term | Hence or otherwise | Question number | 12 | Adapted from | N/A |
Question
In the following diagram, ABCD is the square base of a right pyramid with vertex V. The centre of the base is O. The diagonal of the base, AC, is 8 cm long. The sloping edges are 10 cm long.
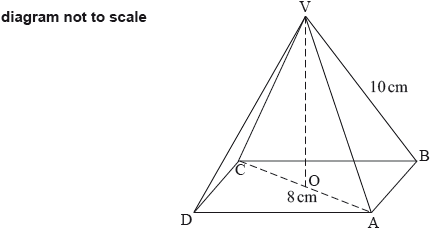
Write down the length of AO.
Find the size of the angle that the sloping edge VA makes with the base of the pyramid.
Hence, or otherwise, find the area of the triangle CAV.
Markscheme
AO=4 (cm) (A1) (C1)
cosO^AV=410 (M1)
Note: Award (M1) for their correct trigonometric ratio.
OR
cosO^AV=102+82−1022×10×8OR102+42−(9.16515…)22×10×4 (M1)
Note: Award (M1) for correct substitution into the cosine rule formula.
O^AV=66.4∘(66.4218…) (A1)(ft) (C2)
Notes: Follow through from their answer to part (a).
area=8×10×sin(66.4218…∘)2OR12×8×√102−42
OR12×10×10×sin(47.1563…∘) (M1)(A1)(ft)
Notes: Award (M1) for substitution into the area formula, (A1)(ft) for correct substitutions. Follow through from their answer to part (b) and/or part (a).
area=36.7 cm2(36.6606… cm2) (A1)(ft) (C3)
Notes: Accept an answer of 8√21 cm2 which is the exact answer.

