| Date | November 2014 | Marks available | 4 | Reference code | 14N.2.sl.TZ0.4 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Calculate | Question number | 4 | Adapted from | N/A |
Question
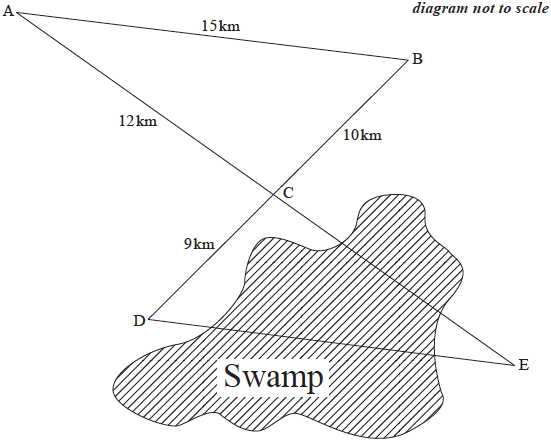
A surveyor has to calculate the area of a triangular piece of land, DCE.
The lengths of CE and DE cannot be directly measured because they go through a swamp.
AB, DE, BD and AE are straight paths. Paths AE and DB intersect at point C.
The length of AB is 15 km, BC is 10 km, AC is 12 km, and DC is 9 km.
The following diagram shows the surveyor’s information.
(i) Find the size of angle ACB.
(ii) Show that the size of angle DCE is 85.5∘, correct to one decimal place.
The surveyor measures the size of angle CDE to be twice that of angle DEC.
(i) Using angle DCE=85.5∘, find the size of angle DEC.
(ii) Find the length of DE.
Calculate the area of triangle DEC.
Markscheme
(i) cosAˆCB=102+122−1522×10×12 (M1)(A1)
Note: Award (M1) for substituted cosine rule,
(A1) for correct substitution.
AˆCB=85.5∘(85.4593…) (A1)(G2)
(ii) DˆCE=AˆCBandAˆCB=85.5∘(85.4593…∘) (A1)
OR
BˆCE=180∘−85.5∘=94.5∘andDˆCE=180∘−94.5∘=85.5∘ (A1)
Notes: Both reasons must be seen for the (A1) to be awarded.
DˆCE=85.5∘ (AG)
(i) DˆEC=180∘−85.5∘3 (M1)
DˆEC=31.5∘ (A1)(G2)
(ii) sin(31.5∘)9=sin(85.5∘)DE (M1)(A1)(ft)
Note: Award (M1) for substituted sine rule, (A1) for correct substitution.
DE=17.2 (km)(17.1718…). (A1)(ft)(G2)
0.5×17.1718…×9×sin(63∘) (A1)(ft)(M1)(A1)(ft)
Note: Award (A1)(ft) for 63 seen, (M1) for substituted triangle area formula, (A1)(ft) for 0.5×17.1718…×9×sin(their angle CDE).
OR
(triangle height=) 9×sin(63∘) (A1)(ft)(A1)(ft)
0.5×17.1718…×9×sin(their angle CDE) (M1)
Note: Award (A1)(ft) for 63 seen, (A1)(ft) for correct triangle height with their angle CDE, (M1) for 0.5×17.1718…×9×sin(their angle CDE).
=68.9 km2(68.8509…) (A1)(ft)(G3)
Notes: Units are required for the last (A1)(ft) mark to be awarded.
Follow through from parts (b)(i) and (b)(ii).
Follow through from their angle CDE within this part of the question.

