| Date | May 2017 | Marks available | 7 | Reference code | 17M.2.sl.TZ1.10 |
| Level | SL only | Paper | 2 | Time zone | TZ1 |
| Command term | Find | Question number | 10 | Adapted from | N/A |
Question
Let f(x)=lnxf(x)=lnx and g(x)=3+ln(x2)g(x)=3+ln(x2), for x>0x>0.
The graph of gg can be obtained from the graph of ff by two transformations:
a horizontal stretch of scale factor q followed bya translation of (hk).a horizontal stretch of scale factor q followed bya translation of (hk).
Let h(x)=g(x)×cos(0.1x)h(x)=g(x)×cos(0.1x), for 0<x<40<x<4. The following diagram shows the graph of hh and the line y=xy=x.
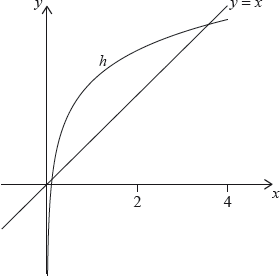
The graph of hh intersects the graph of h−1h−1 at two points. These points have xx coordinates 0.111 and 3.31 correct to three significant figures.
Write down the value of qq;
Write down the value of hh;
Write down the value of kk.
Find ∫3.310.111(h(x)−x)dx∫3.310.111(h(x)−x)dx.
Hence, find the area of the region enclosed by the graphs of hh and h−1h−1.
Let dd be the vertical distance from a point on the graph of hh to the line y=xy=x. There is a point P(a, b)P(a, b) on the graph of hh where dd is a maximum.
Find the coordinates of P, where 0.111<a<3.310.111<a<3.31.
Markscheme
q=2q=2 A1 N1
Note: Accept q=1q=1, h=0h=0, and k=3−ln(2), 2.31 as candidate may have rewritten g(x) as equal to 3+ln(x)−ln(2).
[1 mark]
h=0 A1 N1
Note: Accept q=1, h=0, and k=3−ln(2), 2.31 as candidate may have rewritten g(x) as equal to 3+ln(x)−ln(2).
[1 mark]
k=3 A1 N1
Note: Accept q=1, h=0, and k=3−ln(2), 2.31 as candidate may have rewritten g(x) as equal to 3+ln(x)−ln(2).
[1 mark]
2.72409
2.72 A2 N2
[2 marks]
recognizing area between y=x and h equals 2.72 (M1)
eg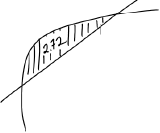
recognizing graphs of h and h−1 are reflections of each other in y=x (M1)
egarea between y=x and h equals between y=x and h−1
2×2.72∫3.310.111(x−h−1(x))dx=2.72
5.44819
5.45 A1 N3
[??? marks]
valid attempt to find d (M1)
egdifference in y-coordinates, d=h(x)−x
correct expression for d (A1)
eg(ln12x+3)(cos0.1x)−x
valid approach to find when d is a maximum (M1)
egmax on sketch of d, attempt to solve d′=0
0.973679
x=0.974 A2 N4
substituting their x value into h(x) (M1)
2.26938
y=2.27 A1 N2
[7 marks]

