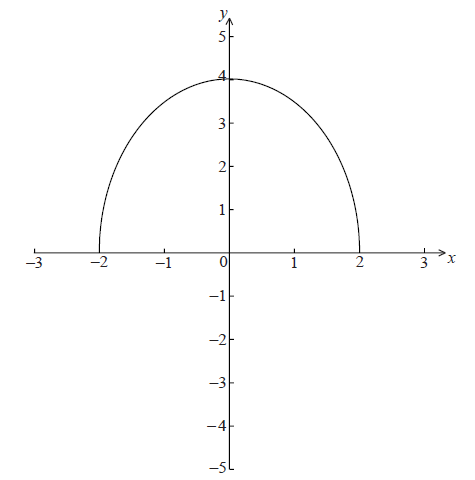
The region enclosed by the curve of f and the x-axis is rotated $360^\circ$ about the x-axis.

Find the volume of the solid formed.

attempt to set up integral expression     M1

e.g. $\pi {\int {\sqrt {16 - 4{x^2}} } ^2}{\rm{d}}x$ , $2\pi \int_0^2 {(16 - 4{x^2})}$ ,  ${\int {\sqrt {16 - 4{x^2}} } ^2}{\rm{d}}x$

$\int {16} {\rm{d}}x = 16x$ , $\int {4{x^2}{\rm{d}}x = } \frac{{4{x^3}}}{3}$ (seen anywhere)     A1A1

evidence of substituting limits into the integrand     (M1)

e.g. $\left( {32 - \frac{{32}}{3}} \right) - \left( { - 32 + \frac{{32}}{3}} \right)$ , $64 - \frac{{64}}{3}$

volume $= \frac{{128\pi }}{3}$     A2     N3

[6 marks]

Many candidates correctly integrated using $f(x)$ , although some neglected to square the function and mired themselves in awkward integration attempts. Upon substituting the limits, many were unable to carry the calculation to completion. Occasionally the $\pi$ was neglected in a final answer. Weaker candidates considered the solid formed to be a sphere and did not use integration.