| Date | May 2017 | Marks available | 6 | Reference code | 17M.2.sl.TZ2.7 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Justify and Find | Question number | 7 | Adapted from | N/A |
Question
Note: In this question, distance is in metres and time is in seconds.
A particle moves along a horizontal line starting at a fixed point A. The velocity v of the particle, at time t, is given by v(t)=2t2−4tt2−2t+2, for 0⩽. The following diagram shows the graph of v
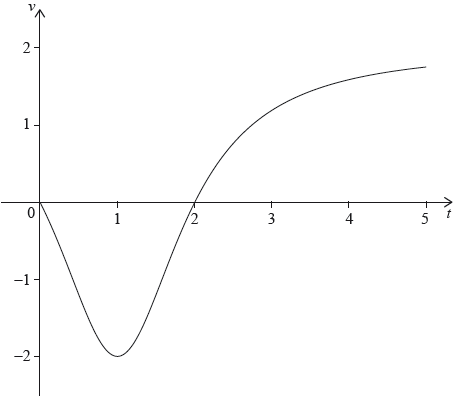
There are t-intercepts at (0,{\text{ }}0) and (2,{\text{ }}0).
Find the maximum distance of the particle from A during the time 0 \leqslant t \leqslant 5 and justify your answer.
Markscheme
METHOD 1 (displacement)
recognizing s = \int {v{\text{d}}t} (M1)
consideration of displacement at t = 2 and t = 5 (seen anywhere) M1
eg\,\,\,\,\,\int_0^2 v and \int_0^5 v
Note: Must have both for any further marks.
correct displacement at t = 2 and t = 5 (seen anywhere) A1A1
- 2.28318 (accept 2.28318), 1.55513
valid reasoning comparing correct displacements R1
eg\,\,\,\,\,\left| { - 2.28} \right| > \left| {1.56} \right|, more left than right
2.28 (m) A1 N1
Note: Do not award the final A1 without the R1.
METHOD 2 (distance travelled)
recognizing distance = \int {\left| v \right|{\text{d}}t} (M1)
consideration of distance travelled from t = 0 to 2 and t = 2 to 5 (seen anywhere) M1
eg\,\,\,\,\,\int_0^2 v and \int_2^5 v
Note: Must have both for any further marks
correct distances travelled (seen anywhere) A1A1
2.28318, (accept - 2.28318), 3.83832
valid reasoning comparing correct distance values R1
eg\,\,\,\,\,3.84 - 2.28 < 2.28,{\text{ }}3.84 < 2 \times 2.28
2.28 (m) A1 N1
Note: Do not award the final A1 without the R1.
[6 marks]

