Find \(\int_1^2 {{{\left( {f(x)} \right)}^2}{\text{d}}x} \).

The following diagram shows part of the graph of \(f\).

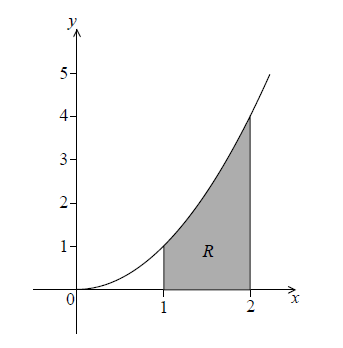
The shaded region \(R\) is enclosed by the graph of \(f\), the \(x\)-axis and the lines \(x = 1\) and \(x = 2\).

Find the volume of the solid formed when \(R\) is revolved \({360^ \circ }\) about the \(x\)-axis.

substituting for \({\left( {f(x)} \right)^2}\) (may be seen in integral)     A1

eg     \({\left( {{x^2}} \right)^2}{\text{, }}{x^4}\)

correct integration, \(\int {{x^4}{\text{d}}x = \frac{1}{5}{x^5}} \)     (A1)

substituting limits into their integrated function and subtracting (in any order)     (M1)

eg     \(\frac{{{2^5}}}{5} - \frac{1}{5}{\text{, }}\frac{1}{5}(1 - 4)\)

\(\int_1^2 {{{\left( {f(x)} \right)}^2}{\text{d}}x} = \frac{{31}}{5}( = 6.2) \)     A1     N2

[4 marks]

attempt to substitute limits or function into formula involving \({f^2}\)     (M1)

eg     \(\int_1^2 {{{\left( {f(x)} \right)}^2}{\text{d}}x{\text{, }}\pi \int {{x^4}{\text{d}}x} } \)

\(\frac{{31}}{5}\pi {\text{   }}( = 6.2\pi )\)     A1     N2

[2 marks]