| Date | May 2017 | Marks available | 2 | Reference code | 17M.2.sl.TZ2.8 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Write down | Question number | 8 | Adapted from | N/A |
Question
Let f(x)=−0.5x4+3x2+2x. The following diagram shows part of the graph of f.
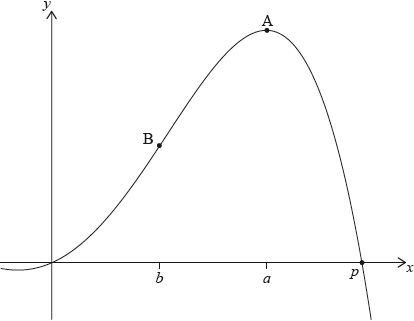
There are x-intercepts at x=0 and at x=p. There is a maximum at A where x=a, and a point of inflexion at B where x=b.
Find the value of p.
Write down the coordinates of A.
Write down the rate of change of f at A.
Find the coordinates of B.
Find the the rate of change of f at B.
Let R be the region enclosed by the graph of f , the x-axis, the line x=b and the line x=a. The region R is rotated 360° about the x-axis. Find the volume of the solid formed.
Markscheme
evidence of valid approach (M1)
egf(x)=0, y=0
2.73205
p=2.73 A1 N2
[2 marks]
1.87938, 8.11721
(1.88, 8.12) A2 N2
[2 marks]
rate of change is 0 (do not accept decimals) A1 N1
[1 marks]
METHOD 1 (using GDC)
valid approach M1
egf″, max/min on f’,{\text{ }}x = - 1
sketch of either f’ or f’’, with max/min or root (respectively) (A1)
x = 1 A1 N1
Substituting their x value into f (M1)
eg\,\,\,\,\,f(1)
y = 4.5 A1 N1
METHOD 2 (analytical)
f’’ = - 6{x^2} + 6 A1
setting f’’ = 0 (M1)
x = 1 A1 N1
substituting their x value into f (M1)
eg\,\,\,\,\,f(1)
y = 4.5 A1 N1
[4 marks]
recognizing rate of change is f’ (M1)
eg\,\,\,\,\,y’,{\text{ }}f’(1)
rate of change is 6 A1 N2
[3 marks]
attempt to substitute either limits or the function into formula (M1)
involving {f^2} (accept absence of \pi and/or {\text{d}}x)
eg\,\,\,\,\,\pi \int {{{( - 0.5{x^4} + 3{x^2} + 2x)}^2}{\text{d}}x,{\text{ }}\int_1^{1.88} {{f^2}} }
128.890
{\text{volume}} = 129 A2 N3
[3 marks]

