| Date | November 2017 | Marks available | 2 | Reference code | 17N.2.sl.TZ0.10 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Show that | Question number | 10 | Adapted from | N/A |
Question
Note: In this question, distance is in millimetres.
Let f(x)=x+asin(x−π2)+a, for x⩾.
The graph of f passes through the origin. Let {{\text{P}}_k} be any point on the graph of f with x-coordinate 2k\pi , where k \in \mathbb{N}. A straight line L passes through all the points {{\text{P}}_k}.
Diagram 1 shows a saw. The length of the toothed edge is the distance AB.
The toothed edge of the saw can be modelled using the graph of f and the line L. Diagram 2 represents this model.
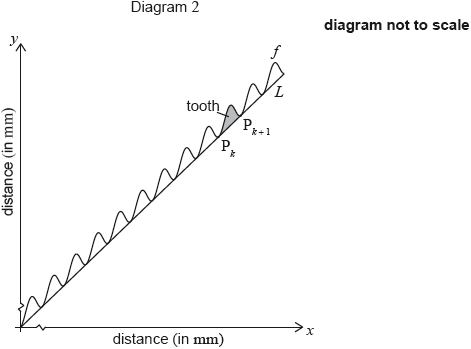
The shaded part on the graph is called a tooth. A tooth is represented by the region enclosed by the graph of f and the line L, between {{\text{P}}_k} and {{\text{P}}_{k + 1}}.
Show that f(2\pi ) = 2\pi .
Find the coordinates of {{\text{P}}_0} and of {{\text{P}}_1}.
Find the equation of L.
Show that the distance between the x-coordinates of {{\text{P}}_k} and {{\text{P}}_{k + 1}} is 2\pi .
A saw has a toothed edge which is 300 mm long. Find the number of complete teeth on this saw.
Markscheme
substituting x = 2\pi M1
eg\,\,\,\,\,2\pi + a\sin \left( {2\pi - \frac{\pi }{2}} \right) + a
2\pi + a\sin \left( {\frac{{3\pi }}{2}} \right) + a (A1)
2\pi - a + a A1
f(2\pi ) = 2\pi AG N0
[3 marks]
substituting the value of k (M1)
{{\text{P}}_0}(0,{\text{ }}0),{\text{ }}{{\text{P}}_1}(2\pi ,{\text{ }}2\pi ) A1A1 N3
[3 marks]
attempt to find the gradient (M1)
eg\,\,\,\,\,\frac{{2\pi - 0}}{{2\pi - 0}},{\text{ }}m = 1
correct working (A1)
eg\,\,\,\,\,\frac{{y - 2\pi }}{{x - 2\pi }} = 1,{\text{ }}b = 0,{\text{ }}y - 0 = 1(x - 0)
y = x A1 N3
[3 marks]
subtracting x-coordinates of {{\text{P}}_{k + 1}} and {{\text{P}}_k} (in any order) (M1)
eg\,\,\,\,\,2(k + 1)\pi - 2k\pi ,{\text{ }}2k\pi - 2k\pi - 2\pi
correct working (must be in correct order) A1
eg\,\,\,\,\,2k\pi + 2\pi - 2k\pi ,{\text{ }}\left| {2k\pi - 2(k + 1)\pi } \right|
distance is 2\pi AG N0
[2 marks]
METHOD 1
recognizing the toothed-edge as the hypotenuse (M1)
eg\,\,\,\,\,{300^2} = {x^2} + {y^2}, sketch
correct working (using their equation of L (A1)
eg\,\,\,\,\,{300^2} = {x^2} + {x^2}
x = \frac{{300}}{{\sqrt 2 }} (exact), 212.132 (A1)
dividing their value of x by 2\pi {\text{ }}\left( {{\text{do not accept }}\frac{{300}}{{2\pi }}} \right) (M1)
eg\,\,\,\,\,\frac{{212.132}}{{2\pi }}
33.7618 (A1)
33 (teeth) A1 N2
METHOD 2
vertical distance of a tooth is 2\pi (may be seen anywhere) (A1)
attempt to find the hypotenuse for one tooth (M1)
eg\,\,\,\,\,{x^2} = {(2\pi )^2} + {(2\pi )^2}
x = \sqrt {8{\pi ^2}} (exact), 8.88576 (A1)
dividing 300 by their value of x (M1)
eg
33.7618 (A1)
33 (teeth) A1 N2
[6 marks]

