| Date | May 2017 | Marks available | 6 | Reference code | 17M.1.SL.TZ1.S_10 |
| Level | Standard Level | Paper | Paper 1 (without calculator) | Time zone | Time zone 1 |
| Command term | Find | Question number | S_10 | Adapted from | N/A |
Question
The following table shows the probability distribution of a discrete random variable A, in terms of an angle θ.
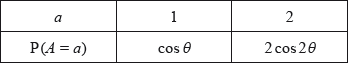
Show that cosθ=34.
Given that tanθ>0, find tanθ.
Let y=1cosx, for 0<x<π2. The graph of ybetween x=θ and x=π4 is rotated 360° about the x-axis. Find the volume of the solid formed.
Markscheme
* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.
evidence of summing to 1 (M1)
eg∑p=1
correct equation A1
egcosθ+2cos2θ=1
correct equation in cosθ A1
egcosθ+2(2cos2θ−1)=1, 4cos2θ+cosθ−3=0
evidence of valid approach to solve quadratic (M1)
egfactorizing equation set equal to 0, −1±√1−4×4×(−3)8
correct working, clearly leading to required answer A1
eg(4cosθ−3)(cosθ+1), −1±78
correct reason for rejecting cosθ≠−1 R1
egcosθ is a probability (value must lie between 0 and 1), cosθ>0
Note: Award R0 for cosθ≠−1 without a reason.
cosθ=34 AG N0
valid approach (M1)
egsketch of right triangle with sides 3 and 4, sin2x+cos2x=1
correct working
(A1)
egmissing side =√7, √7434
tanθ=√73 A1 N2
[3 marks]
attempt to substitute either limits or the function into formula involving f2 (M1)
egπ∫π4θf2, ∫(1cosx)2
correct substitution of both limits and function (A1)
egπ∫π4θ(1cosx)2dx
correct integration (A1)
egtanx
substituting their limits into their integrated function and subtracting (M1)
egtanπ4−tanθ
Note: Award M0 if they substitute into original or differentiated function.
tanπ4=1 (A1)
eg1−tanθ
V=π−π√73 A1 N3
[6 marks]