| Date | November 2017 | Marks available | 6 | Reference code | 17N.1.SL.TZ0.S_8 |
| Level | Standard Level | Paper | Paper 1 (without calculator) | Time zone | Time zone 0 |
| Command term | Find | Question number | S_8 | Adapted from | N/A |
Question
Let f(x)=x2−x, for x∈R. The following diagram shows part of the graph of f.
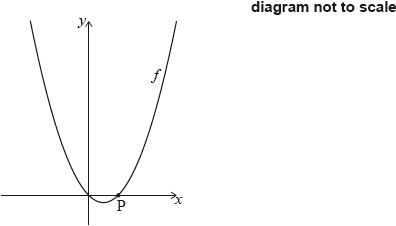
The graph of f crosses the x-axis at the origin and at the point P(1, 0).
The line L intersects the graph of f at another point Q, as shown in the following diagram.
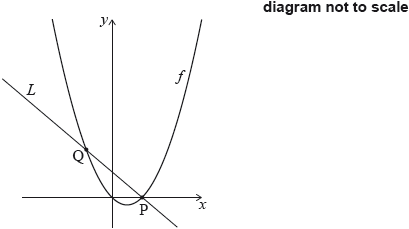
Find the area of the region enclosed by the graph of f and the line L.
Markscheme
valid approach (M1)
eg∫L−f, ∫1−1(1−x2)dx, splitting area into triangles and integrals
correct integration (A1)(A1)
eg[x−x33]1−1, −x33−x22+x22+x
substituting their limits into their integrated function and subtracting (in any order) (M1)
eg1−13−(−1−−13)
Note: Award M0 for substituting into original or differentiated function.
area =43 A2 N3
[6 marks]