| Date | November 2015 | Marks available | 4 | Reference code | 15N.2.sl.TZ0.9 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Find | Question number | 9 | Adapted from | N/A |
Question
An environmental group records the numbers of coyotes and foxes in a wildlife reserve after t years, starting on 1 January 1995.
Let c be the number of coyotes in the reserve after t years. The following table shows the number of coyotes after t years.
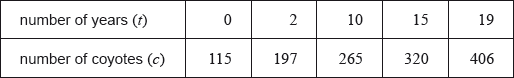
The relationship between the variables can be modelled by the regression equation c=at+b.
Find the value of a and of b.
Use the regression equation to estimate the number of coyotes in the reserve when t=7.
Let f be the number of foxes in the reserve after t years. The number of foxes can be modelled by the equation f=20001+99e−kt, where k is a constant.
Find the number of foxes in the reserve on 1 January 1995.
After five years, there were 64 foxes in the reserve. Find k.
During which year were the number of coyotes the same as the number of foxes?
Markscheme
evidence of setup (M1)
egcorrect value for a or b
13.3823, 137.482
a=13.4, b=137 A1A1 N3
[3 marks]
correct substitution into their regression equation
eg13.3823×7+137.482 (A1)
correct calculation
231.158 (A1)
231 (coyotes) (must be an integer) A1 N2
[3 marks]
recognizing t=0 (M1)
egf(0)
correct substitution into the model
eg20001+99e−k(0), 2000100 (A1)
20 (foxes) A1 N2
[3 marks]
recognizing (5, 64) satisfies the equation (M1)
egf(5)=64
correct substitution into the model
eg64=20001+99e−k(5), 64(1+99\(e^{ - 5k}}) = 2000\) (A1)
0.237124
k=−15ln(1136) (exact), 0.237 [0.237, 0.238] A1 N2
[3 marks]
valid approach (M1)
egc=f, sketch of graphs
correct working (A1)
eg20001+99e−0.237124t=13.382t+137.482, sketch of graphs, table of values
t=12.0403 (A1)
2007 A1 N2
Note: Exception to the FT rule. Award A1FT on their value of t.
[4 marks]
Total [16 marks]

