| Date | None Specimen | Marks available | 3 | Reference code | SPNone.2.sl.TZ0.10 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Find | Question number | 10 | Adapted from | N/A |
Question
A rock falls off the top of a cliff. Let h be its height above ground in metres, after t seconds.
The table below gives values of h and t .

Jane thinks that the function f(t)=−0.25t3−2.32t2+1.93t+106 is a suitable model for the data. Use Jane’s model to
(i) write down the height of the cliff;
(ii) find the height of the rock after 4.5 seconds;
(iii) find after how many seconds the height of the rock is 30 m.
Kevin thinks that the function g(t)=−5.2t2+9.5t+100 is a better model for the data. Use Kevin’s model to find when the rock hits the ground.
(i) On graph paper, using a scale of 1 cm to 1 second, and 1 cm to 10 m, plot the data given in the table.
(ii) By comparing the graphs of f and g with the plotted data, explain which function is a better model for the height of the falling rock.
Markscheme
(i) 106 m A1 N1
(ii) substitute t=4.5 M1
h=44.9 m A1 N2
(iii) set up suitable equation M1
e.g. f(t)=30
t=4.91 A1 N1
[5 marks]
recognizing that height is 0 A1
set up suitable equation M1
e.g. g(t)=0
t=5.39 secs A1 N2
[3 marks]
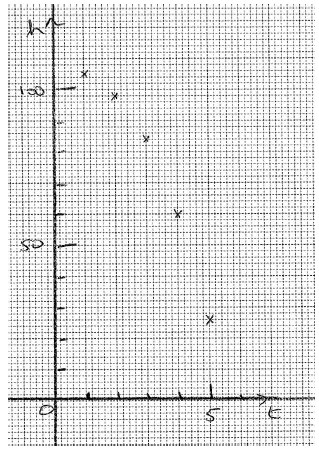 A1A2 N3
A1A2 N3
Note: Award A1 for correct scales on axes, A2 for 5 correct points, A1 for 3 or 4 correct points.
(ii) Jane’s function, with 2 valid reasons A1R1R1 N3
e.g. Jane’s passes very close to all the points, Kevin’s has the rock clearly going up initially – not possible if rock falls
Note: Although Jane’s also goes up initially, it only goes up very slightly, and so is the better model.
[6 marks]

