| Date | November 2011 | Marks available | 4 | Reference code | 11N.2.sl.TZ0.3 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Find | Question number | 3 | Adapted from | N/A |
Question
Consider the following circle with centre O and radius 6.8 cm.
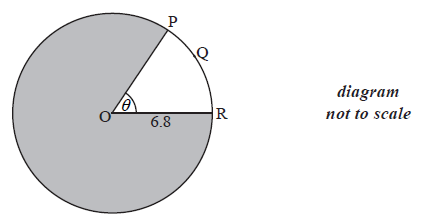
The length of the arc PQR is 8.5 cm.
Find the value of θ .
Find the area of the shaded region.
Markscheme
correct substitution (A1)
e.g. 8.5=θ(6.8) , θ=8.56.8
θ=1.25 (accept 71.6∘ ) A1 N2
[2 marks]
METHOD 1
correct substitution into area formula (seen anywhere) (A1)
e.g. A=π(6.8)2 , 145.267…
correct substitution into area formula (seen anywhere) (A1)
e.g. A=12(1.25)(6.82) , 28.9
valid approach M1
e.g. π(6.8)2−12(1.25)(6.82) ; 145.267…−28.9 ; πr2−12r2sinθ
A=116 (cm2) A1 N2
METHOD 2
attempt to find reflex angle (M1)
e.g. 2π−θ , 360−1.25
correct reflex angle (A1)
AˆOB=2π−1.25 (=5.03318…)
correct substitution into area formula A1
e.g. A=12(5.03318…)(6.82)
A=116 (cm2) A1 N2
[4 marks]
Examiners report
Part (a) was almost universally done correctly.
Many also had little trouble in part (b), with most subtracting from the circle's area, and a minority using the reflex angle. A few candidates worked in degrees, although some of these did so incorrectly by using the radian area formula. Some candidates only found the area of the unshaded sector.

