| Date | May 2015 | Marks available | 4 | Reference code | 15M.2.sl.TZ2.10 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Find, Hence, and Write down | Question number | 10 | Adapted from | N/A |
Question
The following diagram shows a square ABCDABCD, and a sector OABOAB of a circle centre OO, radius rr. Part of the square is shaded and labelled RR.
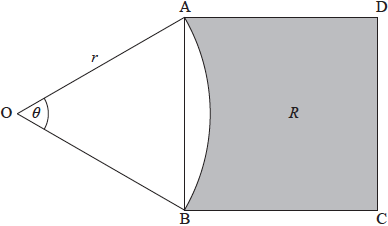
AˆOB=θ, where 0.5 ≤ θ<π.A^OB=θ, where 0.5 ≤ θ<π.
Show that the area of the square ABCDABCD is 2r2(1−cosθ)2r2(1−cosθ).
When θ=αθ=α, the area of the square ABCDABCD is equal to the area of the sector OABOAB.
(i) Write down the area of the sector when θ=αθ=α.
(ii) Hence find αα.
When θ=βθ=β, the area of RR is more than twice the area of the sector.
Find all possible values of ββ.
Markscheme
area of ABCD=AB2ABCD=AB2 (seen anywhere) (A1)
choose cosine rule to find a side of the square (M1)
ega2=b2+c2−2bccosθa2=b2+c2−2bccosθ
correct substitution (for triangle AOBAOB) A1
egr2+r2−2×r×rcosθ, OA2+OB2−2×OA×OBcosθr2+r2−2×r×rcosθ, OA2+OB2−2×OA×OBcosθ
correct working for AB2AB2 A1
eg2r2−2r2cosθ2r2−2r2cosθ
area=2r2(1−cosθ)area=2r2(1−cosθ) AG N0
Note: Award no marks if the only working is 2r2−2r2cosθ2r2−2r2cosθ.
[4 marks]
(i) 12αr2(accept 2r2(1−cosα))12αr2(accept 2r2(1−cosα)) A1 N1
(ii) correct equation in one variable (A1)
eg2(1−cosα)=12α
α=0.511024
α=0.511(accept θ=0.511) A2 N2
Note: Award A1 for α=0.511 and additional answers.
[4 marks]
Note: In this part, accept θ instead of β, and the use of equations instead of inequalities in the working.
attempt to find R (M1)
egsubtraction of areas, square−segment
correct expression for segment area (A1)
eg12βr2−12r2sinβ
correct expression for R (A1)
eg2r2(1−cosβ)−(12βr2−12r2sinβ)
correct inequality (A1)
eg2r2(1−cosβ)−(12βr2−12r2sinβ)>2(12βr2)
correct inequality in terms of angle only A1
eg2(1−cosβ)−(12β−12sinβ)>β
attempt to solve their inequality, must represent R> twice sector (M1)
egsketch, one correct value
Note: Do not award the second (M1) unless the first (M1) for attempting to find R has been awarded.
both correct values 1.30573 and 2.67369 (A1)
correct inequality 1.31<β<2.67 A1 N3
[8 marks]
Total [16 marks]
Examiners report
Those who attempted part (a) could in general show what was required by using the cosine rule. On rare occasions some more complicated approaches were seen using half of angle theta. In some cases, candidates did not show all the necessary steps and lost marks for not completely showing the given result.
A number of candidates correctly answered part (bi) and created a correct equation in (bii), but did not solve the equation correctly, usually attempting an analytic method where the GDC would do. For many a major problem was to realize the need to reduce the equation to one variable before attempting to solve it. Occasionally, an answer would be written that was outside the given domain.
When part (c) was attempted, many candidates did not recognize that the area in question requires the subtraction of a segment area, and often set the square area greater than twice the sector. Many candidates made mistakes when trying to eliminate brackets or just did not use them. Of those who created a correct inequality, few reached a fully correct conclusion.

