The following diagram shows triangle PQR.

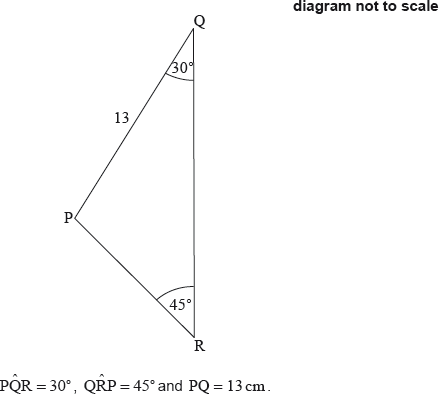
Find PR.

METHOD 1 

evidence of choosing the sine rule     (M1)

eg$\,\,\,\,\,$$\frac{a}{{\sin A}} = \frac{b}{{\sin B}}$

correct substitution     A1

eg$\,\,\,\,\,$$\frac{x}{{\sin 30}} = \frac{{13}}{{\sin 45}},{\text{ }}\frac{{13\sin 30}}{{\sin 45}}$

$\sin 30 = \frac{1}{2},{\text{ }}\sin 45 = \frac{1}{{\sqrt 2 }}$     (A1)(A1)

correct working     A1

eg$\,\,\,\,\,$$\frac{1}{2} \times \frac{{13}}{{\frac{1}{{\sqrt 2 }}}},{\text{ }}\frac{1}{2} \times 13 \times \frac{2}{{\sqrt 2 }},{\text{ }}13 \times \frac{1}{2} \times \sqrt 2$

correct answer     A1     N3

eg$\,\,\,\,\,$${\text{PR}} = \frac{{13\sqrt 2 }}{2},{\text{ }}\frac{{13}}{{\sqrt 2 }}{\text{ (cm)}}$

METHOD 2 (using height of ΔPQR)

valid approach to find height of ΔPQR     (M1)

eg$\,\,\,\,\,$$\sin 30 = \frac{x}{{13}},{\text{ }}\cos 60 = \frac{x}{{13}}$

$\sin 30 = \frac{1}{2}{\text{ or }}\cos 60 = \frac{1}{2}$     (A1)

${\text{height}} = 6.5$     A1

correct working     A1

eg$\,\,\,\,\,$$\sin 45 = \frac{{6.5}}{{{\text{PR}}}},{\text{ }}\sqrt {{{6.5}^2} + {{6.5}^2}}$

correct working     (A1)

eg$\,\,\,\,\,$$\sin 45 = \frac{1}{{\sqrt 2 }},{\text{ }}\cos 45 = \frac{1}{{\sqrt 2 }},{\text{ }}\sqrt {\frac{{169 \times 2}}{4}}$

correct answer     A1     N3

eg$\,\,\,\,\,$${\text{PR}} = \frac{{13\sqrt 2 }}{2},{\text{ }}\frac{{13}}{{\sqrt 2 }}{\text{ (cm)}}$

[6 marks]