| Date | November 2015 | Marks available | 3 | Reference code | 15N.2.sl.TZ0.8 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Find | Question number | 8 | Adapted from | N/A |
Question
The following diagram shows the quadrilateral ABCD.
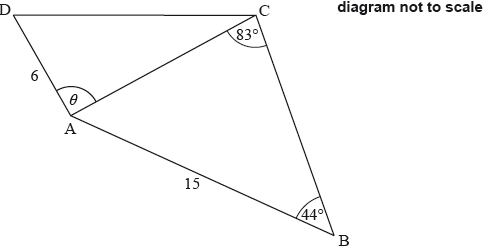
AD=6 cm, AB=15 cm,AˆBC=44∘,AˆCB=83∘andDˆAC=θ
Find AC.
Find the area of triangle ABC.
The area of triangle ACD is half the area of triangle ABC.
Find the possible values of θ.
Given that θ is obtuse, find CD.
Markscheme
evidence of choosing sine rule (M1)
egACsinCˆBA=ABsinAˆCB
correct substitution (A1)
egACsin44∘=15sin83∘
10.4981
AC=10.5 (cm) A1 N2
[3 marks]
finding CˆAB (seen anywhere) (A1)
eg180∘−44∘−83∘,CˆAB=53∘
correct substitution for area of triangle ABC A1
eg12×15×10.4981×sin53∘
62.8813
area=62.9 (cm2) A1 N2
[3 marks]
correct substitution for area of triangle DAC (A1)
eg12×6×10.4981×sinθ
attempt to equate area of triangle ACD to half the area of triangle ABC (M1)
egarea ACD=12× area ABC; 2ACD=ABC
correct equation A1
eg12×6×10.4981×sinθ=12(62.9), 62.9887sinθ=62.8813, sinθ=0.998294
86.6531, 93.3468
θ=86.7∘ , θ=93.3∘ A1A1 N2
[5 marks]
Note: Note: If candidates use an acute angle from part (c) in the cosine rule, award M1A0A0 in part (d).
evidence of choosing cosine rule (M1)
egCD2=AD2+AC2−2×AD×AC×cosθ
correct substitution into rhs (A1)
egCD2=62+10.4982−2(6)(10.498)cos93.336∘
12.3921
12.4 (cm) A1 N2
[3 marks]
Total [14 marks]

