| Date | May 2010 | Marks available | 11 | Reference code | 10M.2.sl.TZ2.6 |
| Level | SL | Paper | 2 | Time zone | TZ2 |
| Command term | Define, Explain, Outline, Sketch, and State | Question number | 6 | Adapted from | N/A |
Question
Water is an important substance that is abundant on the Earth’s surface. Water dissociates according to the following equation.
H2O(l)⇌H+(aq)+OH−(aq)
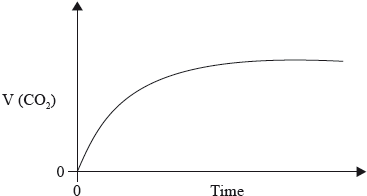
The graph below shows how the volume of carbon dioxide formed varies with time when a hydrochloric acid solution is added to excess calcium carbonate in a flask.
(i) State the equilibrium constant expression for the dissociation of water.
(ii) Explain why even a very acidic aqueous solution still has some OH− ions present in it.
(iii) State and explain the effect of increasing temperature on the equilibrium constant above given that the dissociation of water is an endothermic process.
(iv) The pH of a solution is 2. If its pH is increased to 6, deduce how the hydrogen ion concentration changes.
In carbonated drinks containing dissolved carbon dioxide under high pressure, the
following dynamic equilibrium exists.
CO2(aq)⇌CO2(g)
Describe the effect of opening a carbonated drink container and outline how this
equilibrium is affected.
(i) Explain the shape of the curve.
(ii) Copy the above graph on your answer sheet and sketch the curve you would obtain if double the volume of hydrochloric acid solution of half the concentration as in the example above is used instead, with all other variables kept constant from the original. Explain why the shape of the curve is different.
(iii) Outline one other way in which the rate of this reaction can be studied in a school laboratory. Sketch a graph to illustrate how the selected variable would change with time.
(iv) Define the term activation energy and state one reason why the reaction between calcium carbonate and hydrochloric acid takes place at a reasonably fast rate at room temperature.
Markscheme
(i) Kc=[H+][OH−][H2O]/Kc=[H3O]+[OH−][H2O]/Kw=[H+][OH−]/Kw=[H3O+][OH−];
Do not award mark if [ ] are omitted or other brackets are used.
Expression must be consistent with Kc/Kw.
(ii) [H+] increases, [OH−] decreases but still some present (Kw/Kc constant) / [OH−]
cannot go to zero as equilibrium present / [OH−]=Kw[H+]/Kc[H2O][H+], thus [OH−]
cannot be zero / OWTTE;
Accept equilibrium present.
(iii) (changing T disturbs equilibrium) forward reaction favoured / equilibrium shifts to the right;
to use up (some of the) heat supplied;
(Kw/Kc) increases (as both [H+] and [OH−] increase);
(iv) pH=2, [H+]=0.01 moldm−3 and pH=6, [H+]=10−6 moldm−3/[H+]=10−pH;
[H+] decreased/changed by 10000/10−4;
Award [2] for correct final answer.
CO2(g) /gas escapes / (gas) pressure / CO2 (above liquid) decreases / bubbles (of CO2 gas) form in the liquid;
equilibrium shifts to the right (to replace the lost CO2 gas);
(i) rate = increase in volumetime = slope of graph;
initially/to begin with steeper slope / fastest rate / volume of gas/ CO2 produced faster/quickly as concentration of HCl highest / OWTTE;
as reaction progresses/with time, less steep slope / volume of gas production slows / rate decreases due to less frequent collisions as concentration (of HCl) decreases / OWTTE;
curve flattens/becomes horizontal when HCl used up/consumed (as there are no more H+ ions to collide with the CaCO3 particles);
Each mark requires explanation.
(ii) 
less steep curve;
same maximum volume at later time;
half/lower H+/acid concentration less frequent collisions slower rate;
same amount of HCl, same volume CO2 produced;
(iii) mass loss/of CO2 / mass of flask + content;
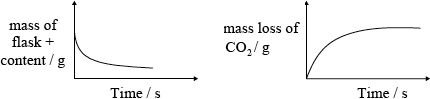 ;
;
OR
 ;
;
OR
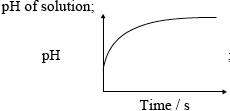 ;
;
Do not penalize for missing x-axis label or for missing units on y-axis.
Accept if line meets time axis.
Award [1 max] if temperature is on the vertical axis and magnitude of slope decreases with time.
(iv) minimum/least energy (of colliding particles) for a reaction to occur / OWTTE;
low/lower Ea /activation energy / greater/larger surface area/contact between CaCO3 and HCl / high/higher HCl concentration/[HCl] / (sufficient) particles/molecules have activation energy;
Examiners report
This was the most popular question in Section B but responses were mixed. Part (a) was generally well dealt with but some candidates confused Kw with Kc or forgot to include charges on the ions in the equilibrium constant expression. Few received the mark for question (ii) although some mentioned equilibrium which was sufficient.
Candidates recognised that increasing the temperature shifts the equilibrium to the right, but most did not explain why, namely to use up some of the heat supplied. The calculation in (iv) was quite well done although some only gave a qualitative answer.
The equilibrium of carbonated drinks was well understood.
In part (c) (i) candidates frequently described the shape of the curve instead of offering an explanation using collisions theory. Candidates did state, for example, that the curve flattens but did not refer to consumption of HCl(aq), the limiting reagent. Only the better candidates were only able to link slope with rate and some still consider the rate to increase after the reaction has started. In (ii) most realised that the curve would be less steep but few drew a curve with the same maximum volume produced at a later time. Even fewer candidates were able to explain why the number of moles of carbon dioxide remained the same. Although some candidates chose mass loss / pH / pressure as the dependant variable in c(iii), some were penalised for imprecise answers such as mass of reactants without referring to mass of flask. Others misunderstood the question and described experiments that they had done with catalysis or described changes with temperature as the dependant variable. (c)(iv) was generally well answered, but again some responses lacked precision; the activation energy is the minimum energy needed for a reaction to occur.

