| Date | May 2015 | Marks available | 5 | Reference code | 15M.1.hl.TZ1.11 |
| Level | HL only | Paper | 1 | Time zone | TZ1 |
| Command term | Find and Justify | Question number | 11 | Adapted from | N/A |
Question
Let y(x)=xe3x, x∈R.
Find dydx.
Prove by induction that dnydxn=n3n−1e3x+x3ne3x for n∈Z+.
Find the coordinates of any local maximum and minimum points on the graph of y(x).
Justify whether any such point is a maximum or a minimum.
Find the coordinates of any points of inflexion on the graph of y(x). Justify whether any such point is a point of inflexion.
Hence sketch the graph of y(x), indicating clearly the points found in parts (c) and (d) and any intercepts with the axes.
Markscheme
dydx=1×e3x+x×3e3x=(e3x+3xe3x) M1A1
[2 marks]
let P(n) be the statement dnydxn=n3n−1e3x+x3ne3x
prove for n=1 M1
LHS of P(1) is dydx which is 1×e3x+x×3e3x and RHS is 30e3x+x31e3x R1
as LHS=RHS, P(1) is true
assume P(k) is true and attempt to prove P(k+1) is true M1
assuming dkydxk=k3k−1e3x+x3ke3x
dk+1ydxk+1=ddx(dkydxk) (M1)
=k3k−1×3e3x+1×3ke3x+x3k×3e3x A1
=(k+1)3ke3x+x3k+1e3x(as required) A1
Note: Can award the A marks independent of the M marks
since P(1) is true and P(k) is true ⇒P(k+1) is true
then (by PMI), P(n) is true (∀n∈Z+) R1
Note: To gain last R1 at least four of the above marks must have been gained.
[7 marks]
e3x+x×3e3x=0⇒1+3x=0⇒x=−13 M1A1
point is (−13, −13e) A1
EITHER
d2ydx2=2×3e3x+x×32e3x
when x=−13, d2ydx2>0 therefore the point is a minimum M1A1
OR

nature table shows point is a minimum M1A1
[5 marks]
d2ydx2=2×3e3x+x×32e3x A1
2×3e3x+x×32e3x=0⇒2+3x=0⇒x=−23 M1A1
point is (−23, −23e2) A1
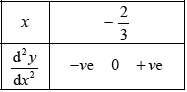
since the curvature does change (concave down to concave up) it is a point of inflection R1
Note: Allow 3rd derivative is not zero at −23
[5 marks]
(general shape including asymptote and through origin) A1
showing minimum and point of inflection A1
Note: Only indication of position of answers to (c) and (d) required, not coordinates.
[2 marks]
Total [21 marks]
Examiners report
Well done.
The logic of an induction proof was not known well enough. Many candidates used what they had to prove rather than differentiating what they had assumed. They did not have enough experience in doing Induction proofs.
Good, some forgot to test for min/max, some forgot to give the y value.
Again quite good, some forgot to check for change in curvature and some forgot the y value.
Some accurate sketches, some had all the information from earlier parts but could not apply it. The asymptote was often missed.

