| Date | November 2013 | Marks available | 1 | Reference code | 13N.1.hl.TZ0.3 |
| Level | HL only | Paper | 1 | Time zone | TZ0 |
| Command term | State | Question number | 3 | Adapted from | N/A |
Question
The diagram below shows a sketch of the graph of y=f(x).
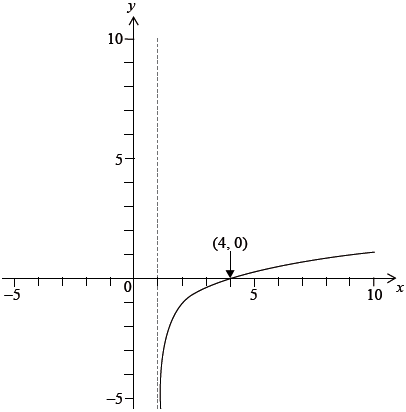
Sketch the graph of y=f−1(x) on the same axes.
State the range of f−1.
Given that f(x)=ln(ax+b), x>1, find the value of a and the value of b.
Markscheme
(a) 
shape with y-axis intercept (0, 4) A1
Note: Accept curve with an asymptote at x=1 suggested.
correct asymptote y=1 A1
[2 marks]
range is f−1(x)>1 (or ]1, ∞[) A1
Note: Also accept ]1, 10] or ]1, 10[.
Note: Do not allow follow through from incorrect asymptote in (a).
[1 mark]
(4, 0)⇒ln(4a+b)=0 M1
⇒4a+b=1 A1
asymptote at x=1⇒a+b=0 M1
⇒a=13, b=−13 A1
[4 marks]
Examiners report
A number of candidates were able to answer a) and b) correctly but found part c) more challenging. Correct sketches for the inverse were seen, but with a few missing a horizontal asymptote. The range in part b) was usually seen correctly. In part c), only a small number of very good candidates were able to gain full marks. A large number used the point (4, 0) to form the equation 4a+b=1 but were unable (or did not recognise the need) to use the asymptote to form a second equation.
A number of candidates were able to answer a) and b) correctly but found part c) more challenging. Correct sketches for the inverse were seen, but with a few missing a horizontal asymptote. The range in part b) was usually seen correctly. In part c), only a small number of very good candidates were able to gain full marks. A large number used the point (4, 0) to form the equation 4a+b=1 but were unable (or did not recognise the need) to use the asymptote to form a second equation.
A number of candidates were able to answer a) and b) correctly but found part c) more challenging. Correct sketches for the inverse were seen, but with a few missing a horizontal asymptote. The range in part b) was usually seen correctly. In part c), only a small number of very good candidates were able to gain full marks. A large number used the point (4, 0) to form the equation 4a+b=1 but were unable (or did not recognise the need) to use the asymptote to form a second equation.

