Sketch the graph of \(y = \frac{{1 - 3x}}{{x - 2}}\), showing clearly any asymptotes and stating the coordinates of any points of intersection with the axes.

Hence or otherwise, solve the inequality \(\left| {\frac{{1 - 3x}}{{x - 2}}} \right| < 2\).

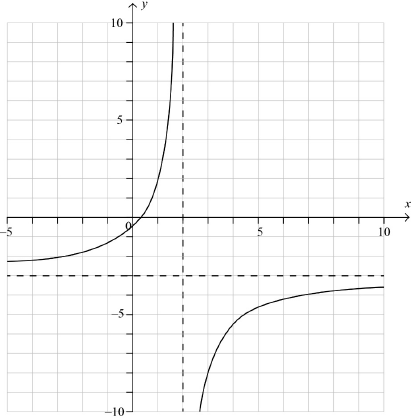
correct vertical asymptote     A1

shape including correct horizontal asymptote     A1

\(\left( {\frac{1}{3},{\text{ }}0} \right)\)     A1

\(\left( {0,{\text{ }} - \frac{1}{2}} \right)\)     A1

 

Note:     Accept \(x = \frac{1}{3}\) and \(y =  - \frac{1}{2}\) marked on the axes.

 

[4 marks]

METHOD 1

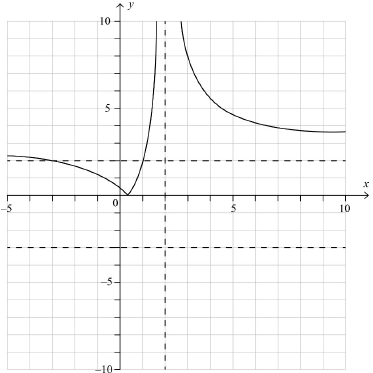
\(\frac{{1 - 3x}}{{x - 2}} = 2\)     (M1)

\( \Rightarrow x = 1\)    A1

\( - \left( {\frac{{1 - 3x}}{{x - 2}}} \right) = 2\)     (M1)

 

Note:     Award this M1 for the line above or a correct sketch identifying a second critical value.

 

\( \Rightarrow x =  - 3\)     A1

solution is \( - 3 < x < 1\)     A1

 

METHOD 2

\(\left| {1 - 3x} \right| < 2\left| {x - 2} \right|,{\text{ }}x \ne 2\)

\(1 - 6x + 9{x^2} < 4({x^2} - 4x + 4)\)     (M1)A1

\(1 - 6x + 9{x^2} < 4{x^2} - 16x + 16\)

\(5{x^2} + 10x - 15 < 0\)

\({x^2} + 2x - 3 < 0\)     A1

\((x + 3)(x - 1) < 0\)     (M1)

solution is \( - 3 < x < 1\)     A1

 

METHOD 3

\( - 2 < \frac{{1 - 3x}}{{x - 2}} < 2\)

consider \(\frac{{1 - 3x}}{{x - 2}} < 2\)     (M1)

 

Note:     Also allow consideration of “>” or “=” for the awarding of the M mark.

 

recognition of critical value at \(x = 1\)     A1

consider \( - 2 < \frac{{1 - 3x}}{{x - 2}}\)     (M1)

 

Note:     Also allow consideration of “>” or “=” for the awarding of the M mark.

 

recognition of critical value at \(x =  - 3\)     A1

solution is \( - 3 < x < 1\)     A1

[5 marks]

 

[N/A]

[N/A]