Let $f(x) = \left| x \right| - 1$.

(a)     The graph of $y = g(x)$ is drawn below.

          (i)     Find the value of $(f \circ g)(1)$.

          (ii)     Find the value of $(f \circ g \circ g)(1)$.

          (iii)     Sketch the graph of $y = (f \circ g)(x)$.

(b)     (i)     Sketch the graph of $y = f(x)$.

          (ii)     State the zeros of f.

(c)     (i)     Sketch the graph of $y = (f \circ f)(x)$.

          (ii)     State the zeros of $f \circ f$.

(d)     Given that we can denote $\underbrace {f \circ f \circ f \circ  \ldots  \circ f}_{n{\text{ times}}}$ as ${f^n}$,

          (i)     find the zeros of ${f^3}$;

          (ii)     find the zeros of ${f^4}$;

          (iii)     deduce the zeros of ${f^8}$.

(e)     The zeros of ${f^{2n}}$ are ${a_1},{\text{ }}{a_2},{\text{ }}{a_3},{\text{ }} \ldots {\text{, }}{a_N}$.

          (i)     State the relation between n and N;

          (ii)     Find, and simplify, an expression for $\sum\limits_{r = 1}^N {\left| {{a_r}} \right|}$ in terms of n.

(a)     (i)     $f(0) =  - 1$     (M1)A1

          (ii)     $(f \circ g)(0) = f(4) = 3$     A1

          (iii)
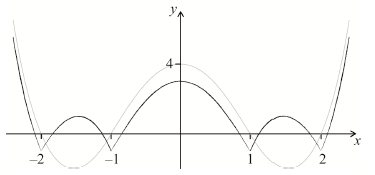               (M1)A1

Note:     Award M1 for evidence that the lower part of the graph has been reflected and A1 correct shape with y-intercept below 4.

[5 marks]

(b)     (i)
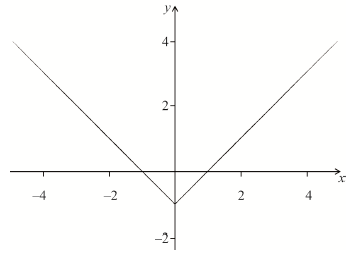               (M1)A1

Note:     Award M1 for any translation of $y = \left| x \right|$.

          (ii)     $\pm 1$     A1

Note:     Do not award the A1 if coordinates given, but do not penalise in the rest of the question

[3 marks]

(c)     (i)
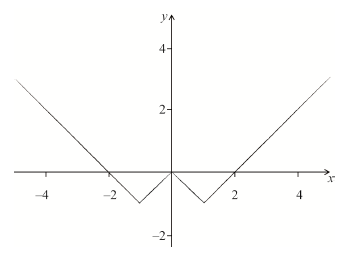               (M1)A1

Note:     Award M1 for evidence that lower part of (b) has been reflected in the x-axis and translated.

          (ii)     $0,{\text{ }} \pm 2$     A1

[3 marks]

(d)     (i)     $\pm 1,{\text{ }} \pm 3$     A1

          (ii)     $0,{\text{ }} \pm 2,{\text{ }} \pm 4$     A1

          (iii)     $0,{\text{ }} \pm 2,{\text{ }} \pm 4,{\text{ }} \pm 6,{\text{ }} \pm 8$     A1

[3 marks]

(e)     (i)     ${\text{(1, 3), (2, 5), }} \ldots$     (M1)

          $N = 2n + 1$     A1

          (ii)     Using the formula of the sum of an arithmetic series     (M1)

          EITHER

          $4(1 + 2 + 3 +  \ldots  + n) = \frac{4}{2}n(n + 1)$

          $= 2n(n + 1)$     A1

          OR

          $2(2 + 4 + 6 +  \ldots  + 2n) = \frac{2}{2}n(2n + 2)$

          $= 2n(n + 1)$     A1

[4 marks]

Total [18 marks]