Express ${x^2} + 3x + 2$ in the form ${(x + h)^2} + k$.

Factorize ${x^2} + 3x + 2$.

Sketch the graph of $f(x)$, indicating on it the equations of the asymptotes, the coordinates of the $y$-intercept and the local maximum.

Show that $\frac{1}{{x + 1}} - \frac{1}{{x + 2}} = \frac{1}{{{x^2} + 3x + 2}}$.

Hence find the value of $p$ if $\int_0^1 {f(x){\text{d}}x = \ln (p)}$.

Sketch the graph of $y = f\left( {\left| x \right|} \right)$.

Determine the area of the region enclosed between the graph of $y = f\left( {\left| x \right|} \right)$, the $x$-axis and the lines with equations $x = - 1$ and $x = 1$.

${x^2} + 3x + 2 = {\left( {x + \frac{3}{2}} \right)^2} - \frac{1}{4}$     A1

[1 mark]

${x^2} + 3x + 2 = (x + 2)(x + 1)$     A1

[1 mark]

A1 for the shape

A1 for the equation $y = 0$

A1 for asymptotes $x = - 2$ and $x = - 1$

A1 for coordinates $\left( { - \frac{3}{2},{\text{ }} - 4} \right)$

A1 $y$-intercept $\left( {0,{\text{ }}\frac{1}{2}} \right)$

[5 marks]

$\frac{1}{{x + 1}} - \frac{1}{{x + 2}} = \frac{{(x + 2) - (x + 1)}}{{(x + 1)(x + 2)}}$     M1

$= \frac{1}{{{x^2} + 3x + 2}}$     AG

[1 mark]

$\int\limits_0^1 {\frac{1}{{x + 1}} - \frac{1}{{x + 2}}{\text{d}}x}$

$= \left[ {\ln (x + 1) - \ln (x + 2)} \right]_0^1$     A1

$= \ln 2 - \ln 3 - \ln 1 + \ln 2$     M1

$= \ln \left( {\frac{4}{3}} \right)$     M1A1

$\therefore p = \frac{4}{3}$

[4 marks]

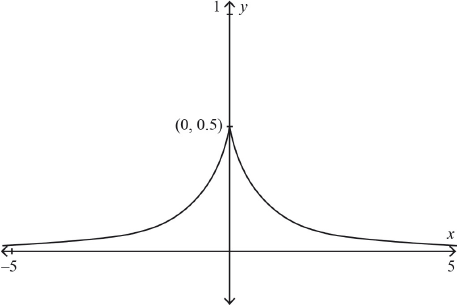
symmetry about the $y$-axis     M1

correct shape     A1

Note:     Allow FT from part (b).

[2 marks]

$2\int_0^1 {f(x){\text{d}}x}$     (M1)(A1)

$= 2\ln \left( {\frac{4}{3}} \right)$     A1

Note:     Do not award FT from part (e).

[3 marks]