| Date | November 2017 | Marks available | 3 | Reference code | 17N.2.hl.TZ0.10 |
| Level | HL only | Paper | 2 | Time zone | TZ0 |
| Command term | Sketch | Question number | 10 | Adapted from | N/A |
Question
Consider the function f(x)=√xsinx, 0<x<π.
Consider the region bounded by the curve y=f(x), the x-axis and the lines x=π6, x=π3.
Show that the x-coordinate of the minimum point on the curve y=f(x) satisfies the equation tanx=2x.
Determine the values of x for which f(x) is a decreasing function.
Sketch the graph of y=f(x) showing clearly the minimum point and any asymptotic behaviour.
Find the coordinates of the point on the graph of f where the normal to the graph is parallel to the line y=−x.
This region is now rotated through 2π radians about the x-axis. Find the volume of revolution.
Markscheme
attempt to use quotient rule or product rule M1
f′(x)=sinx(12x−12)−√xcosxsin2x (=12√xsinx−√xcosxsin2x) A1A1
Note: Award A1 for 12√xsinx or equivalent and A1 for −√xcosxsin2x or equivalent.
setting f′(x)=0 M1
sinx2√x−√xcosx=0
sinx2√x=√xcosx or equivalent A1
tanx=2x AG
[5 marks]
x=1.17
0<x⩽1.17 A1A1
Note: Award A1 for 0<x and A1 for x⩽1.17. Accept x<1.17.
[2 marks]
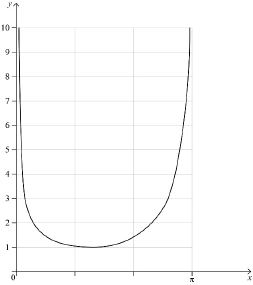
concave up curve over correct domain with one minimum point above the x-axis. A1
approaches x=0 asymptotically A1
approaches x=π asymptotically A1
Note: For the final A1 an asymptote must be seen, and π must be seen on the x-axis or in an equation.
[3 marks]
f′(x) (=sinx(12x−12)−√xcosxsin2x)=1 (A1)
attempt to solve for x (M1)
x=1.96 A1
y=f(1.96…)
=1.51 A1
[4 marks]
V=π∫π3π6xdxsin2x (M1)(A1)
Note: M1 is for an integral of the correct squared function (with or without limits and/or π).
=2.68 (=0.852π) A1
[3 marks]

