| Date | May 2016 | Marks available | 7 | Reference code | 16M.1.hl.TZ1.13 |
| Level | HL only | Paper | 1 | Time zone | TZ1 |
| Command term | Find, Hence, and Prove that | Question number | 13 | Adapted from | N/A |
Question
The following diagram shows the graph of y=(lnx)2x, x>0.
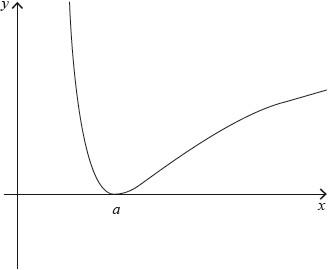
The region R is enclosed by the curve, the x-axis and the line x=e.
Let In=∫e1(lnx)nx2dx, n∈N.
Given that the curve passes through the point (a, 0), state the value of a.
Use the substitution u=lnx to find the area of the region R.
(i) Find the value of I0.
(ii) Prove that In=1e+nIn−1, n∈Z+.
(iii) Hence find the value of I1.
Find the volume of the solid formed when the region R is rotated through 2π about the x-axis.
Markscheme
a=1 A1
[1 mark]
dudx=1x (A1)
∫(lnx)2xdx=∫u2du M1A1
area =[13u3]10 or [13(lnx)3]e1 A1
=13 A1
[5 marks]
(i) I0=[−1x]e1 (A1)
=1−1e A1
(ii) use of integration by parts M1
In=[−1x(lnx)n]e1+∫e1n(lnx)n−1x2dx A1A1
=−1e+nIn−1 AG
Note: If the substitution u=lnx is used A1A1 can be awarded for In=[−e−uun]10+∫10ne−uun−1du.
(iii) I1=−1e+1×I0 (M1)
=1−2e A1
[7 marks]
(d) volume =π∫e1(lnx)4x2dx (=πI4) (A1)
EITHER
I4=−1e+4I3 M1A1
=−1e+4(−1e+3I2) M1
=−5e+12I2=−5e+12(−1e+2I1)
OR
using parts ∫e1(lnx)4x2dx=−1e+4∫e1(lnx)3x2dx M1A1
=−1e+4(−1e+3∫e1(lnx)2x2dx) M1
THEN
=−17e+24(1−2e)=24−65e A1
volume =π(24−65e)
[5 marks]
Examiners report
(a) and (b) were well done. Most candidates could integrate by substitution, though many did not change the limits during the substitution and, though they changed back to x at the end of their solution, under a different markscheme they might have lost marks for this in the intermediate stages.
(a) and (b) were well done. Most candidates could integrate by substitution, though many did not change the limits during the substitution and, though they changed back to x at the end of their solution, under a different markscheme they might have lost marks for this in the intermediate stages.
(c)(i) This part was well done by the candidates.
(c)(ii) This proved to be the part that was done by fewest candidates. Those who spotted that they should use integration by parts obtained the answer fairly easily.
(c)(iii) Many candidates displayed good exam technique in this question and obtained full marks without being able to do part (ii).
The same good exam technique was on show here as many students who failed to prove the expression in (c)(ii) were able to use it to obtain full marks in this question. A few candidates failed to remember correctly the formula for a volume of revolution.

