| Date | May 2013 | Marks available | 5 | Reference code | 13M.2.sl.TZ1.10 |
| Level | SL only | Paper | 2 | Time zone | TZ1 |
| Command term | Find | Question number | 10 | Adapted from | N/A |
Question
A Ferris wheel with diameter 122122 metres rotates clockwise at a constant speed. The wheel completes 2.42.4 rotations every hour. The bottom of the wheel is 1313 metres above the ground.
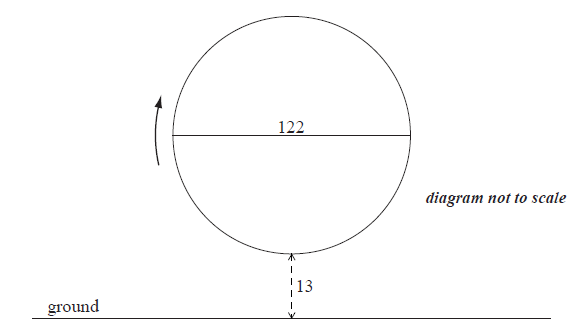
A seat starts at the bottom of the wheel.
After t minutes, the height hh metres above the ground of the seat is given byh=74+acosbt.h=74+acosbt.
Find the maximum height above the ground of the seat.
(i) Show that the period of hh is 2525 minutes.
(ii) Write down the exact value of bb .
(b) (i) Show that the period of hh is 2525 minutes.
(ii) Write down the exact value of bb .
(c) Find the value of aa .
(d) Sketch the graph of hh , for 0≤t≤500≤t≤50 .
Find the value of aa .
Sketch the graph of hh , for 0≤t≤500≤t≤50 .
In one rotation of the wheel, find the probability that a randomly selected seat is at least 105105 metres above the ground.
Markscheme
valid approach (M1)
eg 13+diameter13+diameter , 13+12213+122
maximum height =135=135 (m) A1 N2
[2 marks]
(i) period =602.4=602.4 A1
period =25=25 minutes AG N0
(ii) b=2π25b=2π25 (=0.08π)(=0.08π) A1 N1
[2 marks]
(a) (i) period =602.4=602.4 A1
period =25=25 minutes AG N0
(ii) b=2π25b=2π25 (=0.08π)(=0.08π) A1 N1
[2 marks]
(b) METHOD 1
valid approach (M1)
eg max−74max−74 , |a|=135−132|a|=135−132 , 74−1374−13
|a|=61|a|=61 (accept a=61a=61 ) (A1)
a=−61a=−61 A1 N2
METHOD 2
attempt to substitute valid point into equation for h (M1)
eg 135=74+acos(2π×12.525)135=74+acos(2π×12.525)
correct equation (A1)
eg 135=74+acos(π)135=74+acos(π) , 13=74+a13=74+a
a=−61a=−61 A1 N2
[3 marks]
(c)
A1A1A1A1 N4
Note: Award A1 for approximately correct domain, A1 for approximately correct range,
A1 for approximately correct sinusoidal shape with 22 cycles.
Only if this last A1 awarded, award A1 for max/min in approximately correct positions.
[4 marks]
Total [9 marks]
METHOD 1
valid approach (M1)
eg max−74max−74 , |a|=135−132|a|=135−132 , 74−1374−13
|a|=61|a|=61 (accept a=61a=61 ) (A1)
a=−61a=−61 A1 N2
METHOD 2
attempt to substitute valid point into equation for h (M1)
eg 135=74+acos(2π×12.525)135=74+acos(2π×12.525)
correct equation (A1)
eg 135=74+acos(π)135=74+acos(π) , 13=74+a13=74+a
a=−61a=−61 A1 N2
[3 marks]
A1A1A1A1 N4
Note: Award A1 for approximately correct domain, A1 for approximately correct range,
A1 for approximately correct sinusoidal shape with 22 cycles.
Only if this last A1 awarded, award A1 for max/min in approximately correct positions.
[4 marks]
setting up inequality (accept equation) (M1)
eg h>105h>105 , 105=74+acosbt105=74+acosbt , sketch of graph with line y=105y=105
any two correct values for t (seen anywhere) A1A1
eg t=8.371…t=8.371… , t=16.628…t=16.628… , t=33.371…t=33.371… , t=41.628…t=41.628…
valid approach M1
eg 16.628−8.3712516.628−8.37125 , t1−t225t1−t225 , 2×8.257502×8.25750 , 2(12.5−8.371)252(12.5−8.371)25
p=0.330p=0.330 A1 N2
[5 marks]
Examiners report
Most candidates were successful with part (a).
A surprising number had difficulty producing enough work to show that the period was 2525; writing down the exact value of bb also overwhelmed a number of candidates. In part (c), candidates did not recognize that the seat on the Ferris wheel is a minimum at t=0t=0 thereby making the value of a negative. Incorrect values of 6161 were often seen with correct follow through obtained when sketching the graph in part (d). Graphs again frequently failed to show key features in approximately correct locations and candidates lost marks for incorrect domains and ranges.
A surprising number had difficulty producing enough work to show that the period was 2525; writing down the exact value of bb also overwhelmed a number of candidates. In part (c), candidates did not recognize that the seat on the Ferris wheel is a minimum at t=0t=0 thereby making the value of a negative. Incorrect values of 6161 were often seen with correct follow through obtained when sketching the graph in part (d). Graphs again frequently failed to show key features in approximately correct locations and candidates lost marks for incorrect domains and ranges.
A surprising number had difficulty producing enough work to show that the period was 2525; writing down the exact value of bb also overwhelmed a number of candidates. In part (c), candidates did not recognize that the seat on the Ferris wheel is a minimum at t=0t=0 thereby making the value of a negative. Incorrect values of 6161 were often seen with correct follow through obtained when sketching the graph in part (d). Graphs again frequently failed to show key features in approximately correct locations and candidates lost marks for incorrect domains and ranges.
A surprising number had difficulty producing enough work to show that the period was 2525; writing down the exact value of bb also overwhelmed a number of candidates. In part (c), candidates did not recognize that the seat on the Ferris wheel is a minimum at t=0t=0 thereby making the value of a negative. Incorrect values of 6161 were often seen with correct follow through obtained when sketching the graph in part (d). Graphs again frequently failed to show key features in approximately correct locations and candidates lost marks for incorrect domains and ranges.
Part (e) was very poorly done for those who attempted the question and most did not make the connection between height, time and probability. The idea of linking probability with a real-life scenario proved beyond most candidates. That said, there were a few novel approaches from the strongest of candidates using circles and angles to solve this part of question 10.

