Let $f(x) = 15 - {x^2}$, for $x \in \mathbb{R}$. The following diagram shows part of the graph of $f$ and the rectangle OABC, where A is on the negative $x$-axis, B is on the graph of $f$, and C is on the $y$-axis.

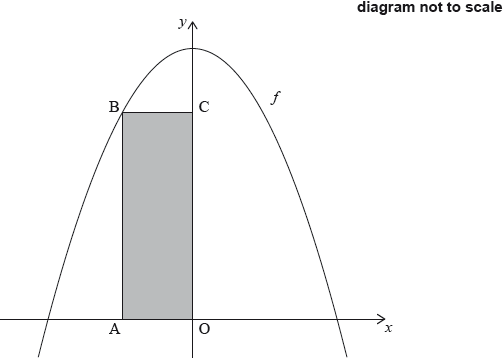
Find the $x$-coordinate of A that gives the maximum area of OABC.

attempt to find the area of OABC     (M1)

eg$\,\,\,\,\,$${\text{OA}} \times {\text{OC, }}x \times f(x),{\text{ }}f(x) \times ( - x)$

correct expression for area in one variable     (A1)

eg$\,\,\,\,\,$${\text{area}} = x(15 - {x^2}),{\text{ }}15x - {x^3},{\text{ }}{x^3} - 15x$

valid approach to find maximum area (seen anywhere)     (M1)

eg$\,\,\,\,\,$$A’(x) = 0$

correct derivative     A1

eg$\,\,\,\,\,$$15 - 3{x^2},{\text{ }}(15 - {x^2}) + x( - 2x) = 0,{\text{ }} - 15 + 3{x^2}$

correct working     (A1)

eg$\,\,\,\,\,$$15 = 3{x^2},{\text{ }}{x^2} = 5,{\text{ }}x = \sqrt 5$

$x =  - \sqrt 5 {\text{ }}\left( {{\text{accept A}}\left( { - \sqrt 5 ,{\text{ }}0} \right)} \right)$     A2     N3

[7 marks]