| Date | May 2016 | Marks available | 4 | Reference code | 16M.1.hl.TZ1.5 |
| Level | HL only | Paper | 1 | Time zone | TZ1 |
| Command term | Find | Question number | 5 | Adapted from | N/A |
Question
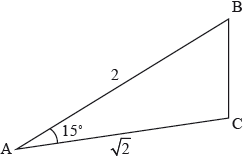
The following diagram shows the triangle ABC where AB=2, AC=√2 and BˆAC=15∘.
Expand and simplify (1−√3)2.
By writing 15∘ as 60∘−45∘ find the value of cos(15∘).
Find BC in the form a+√b where a, b∈Z.
Markscheme
(1−√3)2=4−2√3 A1
Note: Award A0 for 1−2√3+3.
[1 mark]
cos(60∘−45∘)=cos(60∘)cos(45∘)+sin(60∘)sin(45∘) M1
=12×√22+√32×√22 (or 12×1√2+√32×1√2) (A1)
=√2+√64 (or 1+√32√2) A1
[3 marks]
BC2=2+4−2×√2×2cos(15∘) M1
=6−√2(√2+√6)
=4−√12 (=4−2√3) A1
BC=±(1−√3) (M1)
BC=−1+√3 A1
Note: Accept BC=√3−1.
Note: Award M1A0 for 1−√3.
Note: Valid geometrical methods may be seen.
[4 marks]
Examiners report
The main error here was to fail to note the word ‘simplify’ in the question and some candidates wrote 1+3 in their final answer rather than 4.
This was well done by the majority of candidates, though a few wrote cos(60−45)=cos60−cos45.
Candidates were able to use the cosine rule correctly but then failed to notice the result obtained was the same as that obtained in part (a).

