Barry is at the top of a cliff, standing 80 m above sea level, and observes two yachts in the sea.
“Seaview” $(S)$ is at an angle of depression of 25°.
“Nauti Buoy” $(N)$ is at an angle of depression of 35°.
The following three dimensional diagram shows Barry and the two yachts at S and N.
X lies at the foot of the cliff and angle ${\text{SXN}} =$ 70°.

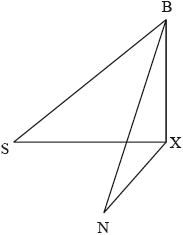
Find, to 3 significant figures, the distance between the two yachts.

attempt to use tan, or sine rule, in triangle BXN or BXS     (M1)

${\text{NX}} = 80\tan 55{\rm{^\circ  }}\left( { = \frac{{80}}{{\tan 35{\rm{^\circ }}}} = 114.25} \right)$     (A1)

${\text{SX}} = 80\tan 65{\rm{^\circ  }}\left( { = \frac{{80}}{{\tan 25{\rm{^\circ }}}} = 171.56} \right)$     (A1)

Attempt to use cosine rule     M1

${\text{S}}{{\text{N}}^2} = {171.56^2} + {114.25^2} - 2 \times 171.56 \times 114.25\cos 70$°     (A1)

${\text{SN}} = 171{\text{ }}({\text{m}})$     A1

Note:     Award final A1 only if the correct answer has been given to 3 significant figures.

[6 marks]