| Date | November 2016 | Marks available | 1 | Reference code | 16N.2.sl.TZ0.2 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Write down | Question number | 2 | Adapted from | N/A |
Question
A group of 66 people went on holiday to Hawaii. During their stay, three trips were arranged: a boat trip (BB), a coach trip (CC) and a helicopter trip (HH).
From this group of people:
| 3 | went on all three trips; |
| 16 | went on the coach trip only; |
| 13 | went on the boat trip only; |
| 5 | went on the helicopter trip only; |
| x | went on the coach trip and the helicopter trip but not the boat trip; |
| 2x | went on the boat trip and the helicopter trip but not the coach trip; |
| 4x | went on the boat trip and the coach trip but not the helicopter trip; |
| 8 | did not go on any of the trips. |
One person in the group is selected at random.
Draw a Venn diagram to represent the given information, using sets labelled BB, CC and HH.
Show that x=3x=3.
Write down the value of n(B∩C)n(B∩C).
Find the probability that this person
(i) went on at most one trip;
(ii) went on the coach trip, given that this person also went on both the helicopter trip and the boat trip.
Markscheme
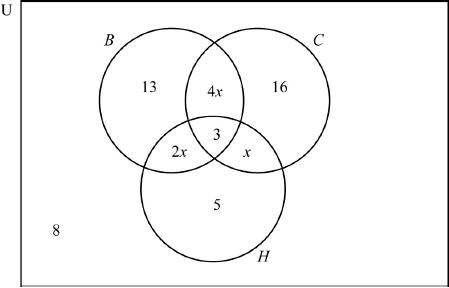 (A5)
(A5)
Notes: Award (A1) for rectangle and three labelled intersecting circles (U need not be seen),
(A1) for 3 in the correct region,
(A1) for 8 in the correct region,
(A1) for 5, 13 and 16 in the correct regions,
(A1) for xx, 2x2x and 4x4x in the correct regions.
[5 marks]
8+13+16+3+5+x+2x+4x=668+13+16+3+5+x+2x+4x=66 (M1)
Note: Award (M1) for either a completely correct equation or adding all the terms from their diagram in part (a) and equating to 66.
Award (M0)(A0) if their equation has no xx.
7x=66−457x=66−45 OR 7x+45=667x+45=66 (A1)
Note: Award (A1) for adding their like terms correctly, but only when the solution to their equation is equal to 3 and is consistent with their original equation.
x=3x=3 (AG)
Note: The conclusion x=3x=3 must be seen for the (A1) to be awarded.
[2 marks]
15 (A1)(ft)
Note: Follow through from part (a). The answer must be an integer.
[1 mark]
(i) 4266 (711, 0.636, 63.6%)4266 (711, 0.636, 63.6%) (A1)(ft)(A1)(G2)
Note: Award (A1)(ft) for numerator, (A1) for denominator. Follow through from their Venn diagram.
(ii) 39 (13, 0.333, 33.3%)39 (13, 0.333, 33.3%) (A1)(A1)(ft)(G2)
Note: Award (A1) for numerator, (A1)(ft) for denominator. Follow through from their Venn diagram.
[4 marks]

