| Date | May 2009 | Marks available | 2 | Reference code | 09M.1.sl.TZ2.9 |
| Level | SL only | Paper | 1 | Time zone | TZ2 |
| Command term | Copy and complete | Question number | 9 | Adapted from | N/A |
Question
Two boxes contain numbered cards as shown below.
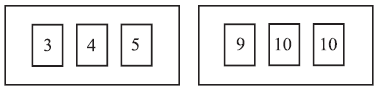
Two cards are drawn at random, one from each box.
Copy and complete the table below to show all nine equally likely outcomes.

Let S be the sum of the numbers on the two cards.
Find the probability of each value of S.
Find the expected value of S.
Anna plays a game where she wins $50$50 if S is even and loses $30 if S is odd.
Anna plays the game 36 times. Find the amount she expects to have at the end of the 36 games.
Markscheme
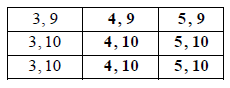 A2 N2
A2 N2
[2 marks]
P(12)=19 , P(13)=39 , P(14)=39 , P(15)=29 A2 N2
[2 marks]
correct substitution into formula for E(X) A1
e.g. E(S)=12×19+13×39+14×39+15×29
E(S)=1239 A2 N2
[3 marks]
METHOD 1
correct expression for expected gain E(A) for 1 game (A1)
e.g. 49×50−59×30
E(A)=509
amount at end = expected gain for 1 game ×36 (M1)
= 200 (dollars) A1 N2
METHOD 2
attempt to find expected number of wins and losses (M1)
e.g. 49×36 , 59×36
attempt to find expected gain E(G) (M1)
e.g. 16×50−30×20
E(G)=200 (dollars) A1 N2
[3 marks]
Examiners report
Most candidates completed parts (a), (b) and (c) successfully.
Most candidates completed part (b) successfully.
Many found the expected value correctly, while some showed difficulty with the arithmetic.
This was often left blank or only superficially attempted. Some found the expected value 509 but did not answer the question about the amount of money.

