| Date | May 2012 | Marks available | 3 | Reference code | 12M.2.sl.TZ1.4 |
| Level | SL only | Paper | 2 | Time zone | TZ1 |
| Command term | Write down | Question number | 4 | Adapted from | N/A |
Question
The graph of y=(x−1)sinxy=(x−1)sinx , for 0≤x≤5π2 , is shown below.
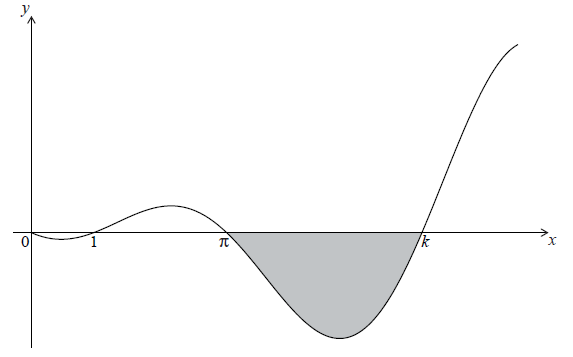
The graph has x-intercepts at 0, 1, π and k .
Find k .
The shaded region is rotated 360∘ about the x-axis. Let V be the volume of the solid formed.
Write down an expression for V .
The shaded region is rotated 360∘ about the x-axis. Let V be the volume of the solid formed.
Find V .
Markscheme
evidence of valid approach (M1)
e.g. y=0 , sinx=0
2π=6.283185…
k=6.28 A1 N2
[2 marks]
attempt to substitute either limits or the function into formula (M1)
(accept absence of dx )
e.g. V=π∫kπ(f(x))2dx , π∫((x−1)sinx)2 , π∫6.28…πy2dx
correct expression A2 N3
e.g. π∫6.28π(x−1)2sin2xdx , π∫2ππ((x−1)sinx)2dx
[3 marks]
V=69.60192562…
V=69.6 A2 N2
[2 marks]
Examiners report
Candidates showed marked improvement in writing fully correct expressions for a volume of revolution. Common errors of course included the omission of dx , using the given domain as the upper and lower bounds of integration, forgetting to square their function and/or the omission of π . There were still many who were unable to use their calculator successfully to find the required volume.
Candidates showed marked improvement in writing fully correct expressions for a volume of revolution. Common errors of course included the omission of dx , using the given domain as the upper and lower bounds of integration, forgetting to square their function and/or the omission of π . There were still many who were unable to use their calculator successfully to find the required volume.
Candidates showed marked improvement in writing fully correct expressions for a volume of revolution. Common errors of course included the omission of dx, using the given domain as the upper and lower bounds of integration, forgetting to square their function and/or the omission of π . There were still many who were unable to use their calculator successfully to find the required volume.

