IB Questionbank

User interface language: English | Español

Date	November 2016	Marks available	6	Reference code	16N.2.SL.TZ0.S_10
Level	Standard Level	Paper	Paper 2	Time zone	Time zone 0
Command term	Hence or otherwise and Find	Question number	S_10	Adapted from	N/A

Question

The following diagram shows the graph of $f(x) = a\sin bx + c$ , for $0 \leqslant x \leqslant 12$ .

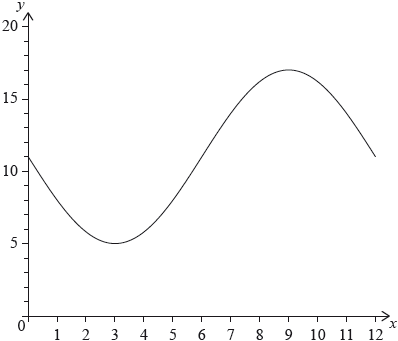
N16/5/MATME/SP2/ENG/TZ0/10

The graph of $f$ has a minimum point at $(3,{\text{ }}5)$ and a maximum point at $(9,{\text{ }}17)$ .

The graph of $g$ is obtained from the graph of $f$ by a translation of $\left( {\begin{array}{*{20}{c}} k \\ 0 \end{array}} \right)$ . The maximum point on the graph of $g$ has coordinates $(11.5,{\text{ }}17)$ .

The graph of $g$ changes from concave-up to concave-down when $x = w$ .

(i) Find the value of $c$ .

(ii) Show that $b = \frac{\pi }{6}$ .

(iii) Find the value of $a$ .

[6]

(i) Write down the value of $k$ .

(ii) Find $g(x)$ .

[3]

(i) Find $w$ .

(ii) Hence or otherwise, find the maximum positive rate of change of $g$ .

[6]

Markscheme

* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.

(i) valid approach (M1)

eg $\,\,\,\,\,$ $\frac{{5 + 17}}{2}$

$c = 11$ A1 N2

(ii) valid approach (M1)

eg $\,\,\,\,\,$ period is 12, per $= \frac{{2\pi }}{b},{\text{ }}9 - 3$

$b = \frac{{2\pi }}{{12}}$ A1

$b = \frac{\pi }{6}$ AG N0

(iii) METHOD 1

valid approach (M1)

eg $\,\,\,\,\,$ $5 = a\sin \left( {\frac{\pi }{6} \times 3} \right) + 11$ , substitution of points

$a = - 6$ A1 N2

METHOD 2

valid approach (M1)

eg $\,\,\,\,\,$ $\frac{{17 - 5}}{2}$ , amplitude is 6

$a = - 6$ A1 N2

[6 marks]

(i) $k = 2.5$ A1 N1

(ii) $g(x) = - 6\sin \left( {\frac{\pi }{6}(x - 2.5)} \right) + 11$ A2 N2

[3 marks]

(i) METHOD 1 Using $g$

recognizing that a point of inflexion is required M1

eg $\,\,\,\,\,$ sketch, recognizing change in concavity

evidence of valid approach (M1)

eg $\,\,\,\,\,$ $g''(x) = 0$ , sketch, coordinates of max/min on ${g'}$

$w = 8.5$ (exact) A1 N2

METHOD 2 Using $f$

recognizing that a point of inflexion is required M1

eg $\,\,\,\,\,$ sketch, recognizing change in concavity

evidence of valid approach involving translation (M1)

eg $\,\,\,\,\,$ $x = w - k$ , sketch, $6 + 2.5$

$w = 8.5$ (exact) A1 N2

(ii) valid approach involving the derivative of $g$ or $f$ (seen anywhere) (M1)

eg $\,\,\,\,\,$ $g'(w),{\text{ }} - \pi \cos \left( {\frac{\pi }{6}x} \right)$ , max on derivative, sketch of derivative

attempt to find max value on derivative M1

eg $\,\,\,\,\,$ $- \pi \cos \left( {\frac{\pi }{6}(8.5 - 2.5)} \right),{\text{ }}f'(6)$ , dot on max of sketch

3.14159

max rate of change $= \pi$ (exact), 3.14 A1 N2

[6 marks]

Examiners report

[N/A]

Syllabus sections

Topic 3— Geometry and trigonometry » SL 3.7—Circular functions: graphs, composites, transformations

Show 89 related questions

19M.2.SL.TZ1.S_8c.i:
Find the value of $b$ and of $c$ .
SPM.2.SL.TZ0.9a:
Find the period of $f$ .
22M.2.SL.TZ2.8b:
Find the values of $t$ when $h_{A} (t) = h_{B} (t)$ .
EXM.3.AHL.TZ0.5a.iii:
Write down the period of this graph
18M.2.SL.TZ1.S_10a:
Find the coordinates of A.
18M.2.SL.TZ1.S_10b.i:
For the graph of $f$ , write down the amplitude.
19M.2.AHL.TZ1.H_10a:
Write down the maximum and minimum value of $v$ .
17M.2.SL.TZ2.S_4c:
Use the model to find the depth of the water 10 hours after high tide.
19M.2.SL.TZ1.S_8b:
Find the range of $g$ .
17N.2.SL.TZ0.S_10a:
Show that $f(2\pi ) = 2\pi$ .
19M.2.AHL.TZ1.H_10c:
Sketch the graph of $y = p\left( t \right)$ for 0 ≤ $t$ ≤ 0.02 , showing clearly the coordinates of the first maximum and the first minimum.
EXM.3.AHL.TZ0.5f.ii:
Show that $\frac{a}{b} = {\text{tan}}\,\theta$ .
17M.2.SL.TZ1.S_8a.i:
How much time is there between the first low tide and the next high tide?
EXM.3.AHL.TZ0.5c.ii:
Comment on your answer to part (c)(i).
EXM.3.AHL.TZ0.5e.i:
$A$ .
EXM.3.AHL.TZ0.5b.ii:
Find the value of $C$ .
17N.2.SL.TZ0.S_10d:
A saw has a toothed edge which is 300 mm long. Find the number of complete teeth on this saw.
EXM.3.AHL.TZ0.5e.iii:
$C$ .
18M.2.SL.TZ1.S_10e:
Find the first time when the ball’s speed is changing at a rate of 2 cm s⁻².
19M.1.AHL.TZ2.H_9a:
Find the $x$ -coordinates of the points of intersection of the two graphs.
19M.2.AHL.TZ1.H_10d:
Find the total time in the interval 0 ≤ $t$ ≤ 0.02 for which $p\left( t \right)$ ≥ 3.
17M.2.SL.TZ1.S_8a.ii:
Find the difference in height between low tide and high tide.
17N.2.SL.TZ0.S_10b.ii:
Find the equation of $L$ .
18M.2.SL.TZ1.S_10d:
Find the maximum speed of the ball.
19M.2.AHL.TZ1.H_10g:
Given that $p\left( t \right)$ can be written as $p\left( t \right) = a\,{\text{sin}}\left( {b\left( {t - c} \right)} \right) + d$ where $a$ , $b$ , $c$ , $d$ > 0, use your graph to find the values of $a$ , $b$ , $c$ and $d$ .
EXM.3.AHL.TZ0.5a.i:
Sketch the graph $y = 3\,{\text{sin}}\,x + 4\,{\text{cos}}\,x$ , for $- 2\pi \leqslant x \leqslant 2\pi$
16N.2.SL.TZ0.S_10a:
(i) Find the value of $c$ .

(ii) Show that $b = \frac{\pi }{6}$ .

(iii) Find the value of $a$ .
19M.1.SL.TZ2.S_7b:
Consider the graph of $g\left( x \right) = 2\,{\text{sin}}\,px$ , 0 ≤ $x$ < $2\pi$ , where $p$ > 0.

Find the greatest value of $p$ such that the graph of $g$ does not intersect the line $y = - 1$ .
EXM.3.AHL.TZ0.5c.i:
Find ${\text{arctan}}\frac{3}{4}$ , giving the answer to 3 significant figures.
EXM.3.AHL.TZ0.5g:
Hence prove your conjectures in part (e).
17N.2.SL.TZ0.S_10b.i:
Find the coordinates of ${{\text{P}}_0}$ and of ${{\text{P}}_1}$ .
22M.1.SL.TZ1.6b:
The $y$ -intercept of the graph of $g$ is at $(0, r)$ .

Given that $g (x) \geq 7$ , find the smallest value of $r$ .
EXN.1.SL.TZ0.9b:
Find the minimum height of the ball above the ground.
EXN.1.SL.TZ0.9d:
For the first 2 seconds of its motion, determine the amount of time that the ball is less than $1.8 + 0.2 \sqrt{2}$ metres above the ground.
17N.2.SL.TZ0.S_10c:
Show that the distance between the $x$ -coordinates of ${{\text{P}}_k}$ and ${{\text{P}}_{k + 1}}$ is $2\pi$ .
19M.2.AHL.TZ1.H_10b:
Write down two transformations that will transform the graph of $y = v\left( t \right)$ onto the graph of $y = i\left( t \right)$ .
22M.2.AHL.TZ2.10b:
Find the values of $t$ when $h_{A} (t) = h_{B} (t)$ .
20N.2.SL.TZ0.S_8a.iii:
Hence find the number of rotations the water wheel makes in one hour.
EXM.3.AHL.TZ0.5b.i:
Use your answers from part (a) to write down the value of $A$ , $B$ and $D$ .
20N.2.SL.TZ0.S_8b:
Find $k$ .
20N.2.SL.TZ0.S_8d:
Determine the rate of change of $h$ when the top of the bucket is at $B$ .
EXM.3.AHL.TZ0.5f.i:
Show that $\frac{b}{{\sqrt {{a^2} + {b^2}} }} = {\text{cos}}\,\theta$ .
19M.2.SL.TZ1.S_8c.ii:
Find the period of $g$ .
19M.2.SL.TZ1.S_8d:
The equation $g\left( x \right) = 12$ has two solutions where $\pi$ ≤ $x$ ≤ ${\frac{{4\pi }}{3}}$ . Find both solutions.
18M.2.SL.TZ1.S_10b.ii:
For the graph of $f$ , write down the period.
19M.1.AHL.TZ2.H_9c:
At the points A and B on the diagram, the gradients of the two graphs are equal.

Determine the $y$ -coordinate of A on the graph of $g$ .
17M.2.SL.TZ2.S_4a:
Find the value of $p$ .
SPM.2.SL.TZ0.9b.ii:
Hence, find the value of $f$ (6).
21M.1.SL.TZ2.8a:
Find the $x$ -coordinates of $A$ and $B$ .
19M.1.AHL.TZ2.H_9b:
Find the exact area of the shaded region, giving your answer in the form $p\pi + q\sqrt 3$ , where $p$ , $q \in \mathbb{Q}$ .
17M.2.SL.TZ1.S_8b.ii:
Find the value of $q$ ;
SPM.2.SL.TZ0.9b.i:
Find the value of $b$ .
EXN.1.SL.TZ0.9a:
Find the height of the ball above the ground when it is released.
EXN.1.SL.TZ0.9c:
Show that the ball takes $2$ seconds to return to its initial height above the ground for the first time.
20N.2.SL.TZ0.S_8c:
Find $A \hat{O} B$ .
19M.2.AHL.TZ1.H_10e:
Find ${p_{av}}$ (0.007).
20N.2.SL.TZ0.S_8a.ii:
Calculate the number of seconds it takes for the water wheel to complete one rotation.
20N.2.SL.TZ0.S_8a.i:
Find the height of point $A$ above the ground.
19M.2.AHL.TZ1.H_10f:
With reference to your graph of $y = p\left( t \right)$ explain why ${p_{av}}\left( T \right)$ > 0 for all $T$ > 0.
17M.2.SL.TZ2.S_4b:
Find the value of $q$ .
19M.2.SL.TZ1.S_8a:
The range of $f$ is $k$ ≤ $f\left( x \right)$ ≤ $m$ . Find $k$ and $m$ .
21M.2.SL.TZ2.7d:
Find the value of $p$ and the value of $q$ .
21M.2.SL.TZ2.7e:
If the observer stands directly under point $C$ for one minute, point $D$ will pass over his head $n$ times.

Find the value of $n$ .
21N.2.SL.TZ0.8a:
Show that $b = \frac{π}{6}$ .
21N.2.SL.TZ0.8c:
Find the value of $d$ .
21N.2.SL.TZ0.8d:
Find the smallest possible value of $c$ .
22M.1.SL.TZ1.6a:
Describe these two transformations.
21N.2.SL.TZ0.8b:
Find the value of $a$ .
21N.2.AHL.TZ0.9a:
Show that $b = \frac{π}{6}$ .
21N.2.AHL.TZ0.9b:
Find the value of $a$ .
21N.2.AHL.TZ0.9d:
Find the smallest possible value of $c$ .
EXM.3.AHL.TZ0.5a.ii:
Write down the amplitude of this graph
EXM.3.AHL.TZ0.5d:
By considering the graph of $y = 5\,{\text{sin}}\,x + 12\,{\text{cos}}\,x$ , find the value of $A$ , $B$ , $C$ and $D$ .
18M.2.SL.TZ1.S_10c:
Hence, write $f\left( x \right)$ in the form $p\,\,{\text{cos}}\,\left( {x + r} \right)$ .
17M.2.SL.TZ1.S_8b.i:
Find the value of $p$ ;
EXM.3.AHL.TZ0.5e.ii:
$B$ .
EXM.3.AHL.TZ0.5e.iv:
$D$ .
17M.2.SL.TZ1.S_8b.iii:
Find the value of $r$ .
17M.2.SL.TZ1.S_8c:
There are two high tides on 12 December 2017. At what time does the second high tide occur?
16N.2.SL.TZ0.S_10b:
(i) Write down the value of $k$ .

(ii) Find $g(x)$ .
SPM.2.SL.TZ0.9d:
Find the value of $x$ for which the functions have the greatest difference.
SPM.2.SL.TZ0.9c:
Find the value of $p$ and the value of $q$ .
21M.2.SL.TZ1.4:
A Ferris wheel with diameter $110$ metres rotates at a constant speed. The lowest point on the wheel is $10$ metres above the ground, as shown on the following diagram. $P$ is a point on the wheel. The wheel starts moving with $P$ at the lowest point and completes one revolution in $20$ minutes.

The height, $h$ metres, of $P$ above the ground after $t$ minutes is given by $h (t) = a \cos (b t) + c$ , where $a, b, c \in ℝ$ .

Find the values of $a$ , $b$ and $c$ .
21N.2.AHL.TZ0.9e:
Find the height of the water at $12 : 00$ .
21N.2.SL.TZ0.8e:
Find the height of the water at $12 : 00$ .
21N.2.SL.TZ0.8f:
Determine the number of hours, over a 24-hour period, for which the tide is higher than $5$ metres.
21N.2.AHL.TZ0.9c:
Find the value of $d$ .
21N.2.AHL.TZ0.9f:
Determine the number of hours, over a 24-hour period, for which the tide is higher than $5$ metres.
21N.2.AHL.TZ0.9g:
A fisherman notes that the water height at nearby Folkestone harbour follows the same sinusoidal pattern as that of Dungeness harbour, with the exception that high tides (and low tides) occur $50$ minutes earlier than at Dungeness.

Find a suitable equation that may be used to model the tidal height of water at Folkestone harbour.

Hide related questions

Topic 3— Geometry and trigonometry

Question

Markscheme

Examiners report

Syllabus sections

View options