| Date | November 2009 | Marks available | 2 | Reference code | 09N.2.sl.TZ0.1 |
| Level | SL | Paper | 2 | Time zone | TZ0 |
| Command term | Identify | Question number | 1 | Adapted from | N/A |
Question
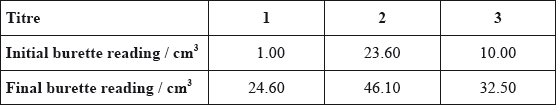
The data below is from an experiment used to determine the percentage of iron present in a sample of iron ore. This sample was dissolved in acid and all of the iron was converted to \({\text{F}}{{\text{e}}^{{\text{2 + }}}}\). The resulting solution was titrated with a standard solution of potassium manganate(VII), \({\text{KMn}}{{\text{O}}_{\text{4}}}\). This procedure was carried out three times. In acidic solution, \({\text{MnO}}_4^ - \) reacts with \({\text{F}}{{\text{e}}^{2 + }}\) ions to form \({\text{M}}{{\text{n}}^{2 + }}\) and \({\text{F}}{{\text{e}}^{3 + }}\) and the end point is indicated by a slight pink colour.
Deduce the balanced redox equation for this reaction in acidic solution.
Identify the reducing agent in the reaction.
Calculate the amount, in moles, of \({\text{MnO}}_4^ - \) used in the titration.
Calculate the amount, in moles, of Fe present in the \(3.682 \times {10^{ - 1}}{\text{ g}}\) sample of iron ore.
Determine the percentage by mass of Fe present in the \(3.682 \times {10^{ - 1}}{\text{ g}}\) sample of iron ore.
Markscheme
\[{\text{MnO}}_4^ - {\text{(aq)}} + {\text{5F}}{{\text{e}}^{2 + }}{\text{(aq)}} + {\text{8}}{{\text{H}}^ + }{\text{(aq)}} \to {\text{M}}{{\text{n}}^{2 + }}{\text{(aq)}} + {\text{5F}}{{\text{e}}^{3 + }}{\text{(aq)}} + {\text{4}}{{\text{H}}_2}{\text{O(l)}}\]
Award [2] if correctly balanced.
Award [1] for correctly placing H+ and H2O.
Award [1 max] for correct balanced equation but with electrons shown.
Ignore state symbols.
\({\text{F}}{{\text{e}}^{2 + }}\) / iron(II);
Do not accept iron.
\({\text{n}} = 2.152 \times {10^{ - 2}} \times 2.250 \times {10^{ - 2}}\);
\(4.842 \times {10^{ - 4}}{\text{ (mol)}}\);
Award [1] for correct volume
Award [1] for correct calculation.
1 mol of \({\text{MnO}}_4^ - \) reacts with 5 mol of \({\text{F}}{{\text{e}}^{2 + }}\);
\(5 \times 4.842 \times {10^{ - 4}} = 2.421 \times {10^{ - 3}}{\text{ (mol)}}\);
(same number of moles of Fe in the iron ore)
Allow ECF from part (a) and (c) provided some mention of mole ratio is stated.
\(2.421 \times {10^{ - 3}} \times 55.85 = 0.1352{\text{ (g)}}\);
\(\frac{{0.1352}}{{0.3682}} \times 100 = 36.72\% \);
Allow ECF from part (d).
Examiners report
Most G2 comments on Section A were about this question. Many commented that titration is not part of the SL syllabus; however it is the expectation that students would cover this and other basic chemical techniques as part of their practical programme. Question 1 in all papers is meant to be data response and students will be expected to be familiar with experimental techniques. Also, there was some confusion caused because there was one sample and three titres. However this unfortunate cause of confusion did not seem to impact on candidate performance as poor performance was found throughout the question even with some very routine questions. Generally this question was poorly answered. In a) not many candidates managed to write the correct balanced equation, however many gave the correct species that were missing, \({{\text{H}}^ + }\) and \({{\text{H}}_{\text{2}}}{\text{O}}\).
Most candidates were able to identify the reducing agent although a few candidates just mentioned “iron” or Fe, but metallic iron was not in the equation.
In (c) candidates were not familiar with the process of selecting the 2 best titres and averaging them. Some chose the first written, some averaged all three and some weaker candidates merely added the 3 titres and used this. Some candidates also forgot to convert cm3 to dm3.
In 1(e), a few candidates scored ECF marks for the % based on n(Fe) calculated in (d). A couple of candidates realised that their answer to (d) did not help and followed on from (c) to find the number of moles of \({\text{F}}{{\text{e}}^{2 + }}\) and hence the % of Fe, scoring ECF marks.
