| Date | May 2008 | Marks available | 4 | Reference code | 08M.2.sl.TZ2.4 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Find | Question number | 4 | Adapted from | N/A |
Question
The following table shows the probability distribution of a discrete random variable X.
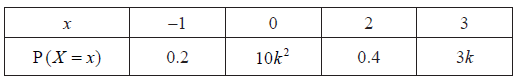
Find the value of k.
Find the expected value of X.
Markscheme
evidence of using ∑pi=1 (M1)
correct substitution A1
e.g. 10k2+3k+0.6=1 , 10k2+3k−0.4=0
k=0.1 A2 N2
[4 marks]
evidence of using E(X)=∑pixi (M1)
correct substitution (A1)
e.g. −1×0.2+2×0.4+3×0.3
E(X)=1.5 A1 N2
[3 marks]
Examiners report
A good number of candidates answered this question well, although some incorrectly set the sum of the probabilities to zero instead of one, suggesting rote recognition of a quadratic equal to zero. Many candidates recognized that only the positive value for k was appropriate and correctly indicated this in their working. Many went on to find the correct expected value as well, although at times candidates wrote the formula from the information booklet without making use of it, thus earning no marks.
A good number of candidates answered this question well, although some incorrectly set the sum of the probabilities to zero instead of one, suggesting rote recognition of a quadratic equal to zero. Many candidates recognized that only the positive value for k was appropriate and correctly indicated this in their working. Many went on to find the correct expected value as well, although at times candidates wrote the formula from the information booklet without making use of it, thus earning no marks.

