| Date | November 2017 | Marks available | 3 | Reference code | 17N.2.sl.TZ0.4 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Find | Question number | 4 | Adapted from | N/A |
Question
A discrete random variable X has the following probability distribution.
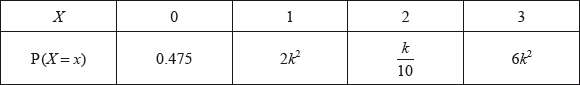
Find the value of k.
[4]
a.
Write down P(X=2).
[1]
b.
Find P(X=2|X>0).
[3]
c.
Markscheme
valid approach (M1)
egtotal probability = 1
correct equation (A1)
eg0.475+2k2+k10+6k2=1, 8k2+0.1k−0.525=0
k=0.25 A2 N3
[4 marks]
a.
P(X=2)=0.025 A1 N1
[1 mark]
b.
valid approach for finding P(X>0) (M1)
eg1−0.475, 2(0.252)+0.025+6(0.252), 1−P(X=0), 2k2+k10+6k2
correct substitution into formula for conditional probability (A1)
eg0.0251−0.475, 0.0250.525
0.0476190
P(X=2|X>0)=121 (exact), 0.0476 A1 N2
[3 marks]
c.
Examiners report
[N/A]
a.
[N/A]
b.
[N/A]
c.
Syllabus sections
Topic 5 - Statistics and probability » 5.7 » Concept of discrete random variables and their probability distributions.
Show 37 related questions

