| Date | May 2012 | Marks available | 2 | Reference code | 12M.1.sl.TZ2.4 |
| Level | SL only | Paper | 1 | Time zone | TZ2 |
| Command term | Find | Question number | 4 | Adapted from | N/A |
Question
The random variable X has the following probability distribution, with P(X>1)=0.5P(X>1)=0.5 .
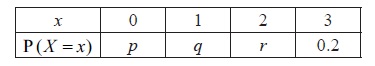
Find the value of r .
Given that E(X)=1.4E(X)=1.4 , find the value of p and of q .
Markscheme
attempt to substitute P(X>1)=0.5P(X>1)=0.5 (M1)
e.g. r+0.2=0.5r+0.2=0.5
r=0.3r=0.3 A1 N2
[2 marks]
correct substitution into E(X)E(X) (seen anywhere) (A1)
e.g. 0×p+1×q+2×r+3×0.20×p+1×q+2×r+3×0.2
correct equation A1
e.g. q+2×0.3+3×0.2=1.4q+2×0.3+3×0.2=1.4 , q+1.2=1.4q+1.2=1.4
q=0.2q=0.2 A1 N1
evidence of choosing ∑pi=1∑pi=1 M1
e.g. p+0.2+0.3+0.2=1p+0.2+0.3+0.2=1 , p+q=0.5p+q=0.5
correct working (A1)
p+0.7=1p+0.7=1 , 1−0.2−0.3−0.21−0.2−0.3−0.2 , p+0.2=0.5p+0.2=0.5
p=0.3p=0.3 A1 N2
Note: Exception to the FT rule. Award FT marks on an incorrect value of q, even if q is an inappropriate value. Do not award the final A mark for an inappropriate value of p.
[6 marks]
Examiners report
The majority of candidates were successful in earning full marks on this question.
In part (b), a small number of candidates did not use the correct formula for E(X)E(X) , even though this formula is given in the formula booklet. There were also a few candidates who incorrectly assumed that p=0p=0 , forgetting that the sum of the probabilities must equal 1. There were a few candidates who left this question blank, which raises concerns about whether they had been exposed to probability distributions during the course.

