| Date | May 2011 | Marks available | 3 | Reference code | 11M.2.sl.TZ2.9 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Find | Question number | 9 | Adapted from | N/A |
Question
Two fair 4-sided dice, one red and one green, are thrown. For each die, the faces are labelled 1, 2, 3, 4. The score for each die is the number which lands face down.
List the pairs of scores that give a sum of 6.
The probability distribution for the sum of the scores on the two dice is shown below.
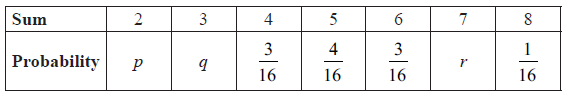
Find the value of p , of q , and of r .
Fred plays a game. He throws two fair 4-sided dice four times. He wins a prize if the sum is 5 on three or more throws.
Find the probability that Fred wins a prize.
Markscheme
three correct pairs A1A1A1 N3
e.g. (2, 4), (3, 3), (4, 2) , R2G4, R3G3, R4G2
[3 marks]
p=116 , q=216 , r=216 A1A1A1 N3
[3 marks]
let X be the number of times the sum of the dice is 5
evidence of valid approach (M1)
e.g. X∼B(n, p) , tree diagram, 5 sets of outcomes produce a win
one correct parameter (A1)
e.g. n=4 , p=0.25 , q=0.75
Fred wins prize is P(X≥3) (A1)
appropriate approach to find probability M1
e.g. complement, summing probabilities, using a CDF function
correct substitution (A1)
e.g. 1−0.949… , 1−243256 , 0.046875+0.00390625 , 12256+1256
probability of winning=0.0508 (13256) A1 N3
[6 marks]
Examiners report
All but the weakest candidates managed to score full marks for parts (a) and (b). An occasional error in part (a) was including additional pair(s) or listing (3, 3) twice.
All but the weakest candidates managed to score full marks for parts (a) and (b).
Many candidates found part (c) challenging, as they failed to recognize the binomial probability. Successful candidates generally used either the binomial CDF function or the sum of two binomial probabilities. Some used approaches like multiplying probabilities or tree diagrams, but these were less successful.

