The following diagram shows [AB], with length 2 cm. The line is divided into an infinite number of line segments. The diagram shows the first three segments.

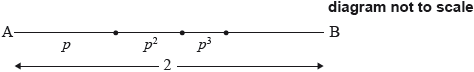
The length of the line segments are \(p{\text{ cm}},{\text{ }}{p^2}{\text{ cm}},{\text{ }}{p^3}{\text{ cm}},{\text{ }} \ldots \), where \(0 < p < 1\).

Show that \(p = \frac{2}{3}\).

The following diagram shows [CD], with length \(b{\text{ cm}}\), where \(b > 1\). Squares with side lengths \(k{\text{ cm}},{\text{ }}{k^2}{\text{ cm}},{\text{ }}{k^3}{\text{ cm}},{\text{ }} \ldots \), where \(0 < k < 1\), are drawn along [CD]. This process is carried on indefinitely. The diagram shows the first three squares.

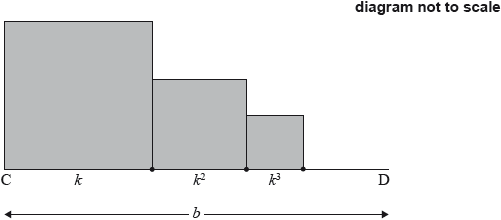
The total sum of the areas of all the squares is \(\frac{9}{{16}}\). Find the value of \(b\).

infinite sum of segments is 2 (seen anywhere)     (A1)

eg\(\,\,\,\,\,\)\(p + {p^2} + {p^3} +  \ldots  = 2,{\text{ }}\frac{{{u_1}}}{{1 - r}} = 2\)

recognizing GP     (M1)

eg\(\,\,\,\,\,\)ratio is \(p,{\text{ }}\frac{{{u_1}}}{{1 - r}},{\text{ }}{u_n} = {u_1} \times {r^{n - 1}},{\text{ }}\frac{{{u_1}({r^n} - 1)}}{{r - 1}}\)

correct substitution into \({S_\infty }\) formula (may be seen in equation)     A1

eg\(\,\,\,\,\,\)\(\frac{p}{{1 - p}}\)

correct equation     (A1)

eg\(\,\,\,\,\,\)\(\frac{p}{{1 - p}} = 2,{\text{ }}p = 2 - 2p\)

correct working leading to answer     A1

eg\(\,\,\,\,\,\)\(3p = 2,{\text{ }}2 - 3p = 0\)

\(p = \frac{2}{3}{\text{ (cm)}}\)     AG     N0

[5 marks]

recognizing infinite geometric series with squares     (M1)

eg\(\,\,\,\,\,\)\({k^2} + {k^4} + {k^6} +  \ldots ,{\text{ }}\frac{{{k^2}}}{{1 - {k^2}}}\)

correct substitution into \({S_\infty } = \frac{9}{{16}}\) (must substitute into formula)     (A2)

eg\(\,\,\,\,\,\)\(\frac{{{k^2}}}{{1 - {k^2}}} = \frac{9}{{16}}\)

correct working     (A1)

eg\(\,\,\,\,\,\)\(16{k^2} = 9 - 9{k^2},{\text{ }}25{k^2} = 9,{\text{ }}{k^2} = \frac{9}{{25}}\)

\(k = \frac{3}{5}\) (seen anywhere)     A1

valid approach with segments and CD (may be seen earlier)     (M1)

eg\(\,\,\,\,\,\)\(r = k,{\text{ }}{S_\infty } = b\)

correct expression for \(b\) in terms of \(k\) (may be seen earlier)     (A1)

eg\(\,\,\,\,\,\)\(b = \frac{k}{{1 - k}},{\text{ }}b = \sum\limits_{n = 1}^\infty  {{k^n},{\text{ }}b = k + {k^2} + {k^3} +  \ldots } \)

substituting their value of \(k\) into their formula for \(b\)     (M1)

eg\(\,\,\,\,\,\)\(\frac{{\frac{3}{5}}}{{1 - \frac{3}{5}}},{\text{ }}\frac{{\left( {\frac{3}{5}} \right)}}{{\left( {\frac{2}{5}} \right)}}\)

\(b = \frac{3}{2}\)     A1     N3

[9 marks]