| Date | May 2014 | Marks available | 7 | Reference code | 14M.1.sl.TZ1.10 |
| Level | SL only | Paper | 1 | Time zone | TZ1 |
| Command term | Find | Question number | 10 | Adapted from | N/A |
Question
The sides of a square are 16 cm in length. The midpoints of the sides of this square are joined to form a new square and four triangles (diagram 1). The process is repeated twice, as shown in diagrams 2 and 3.
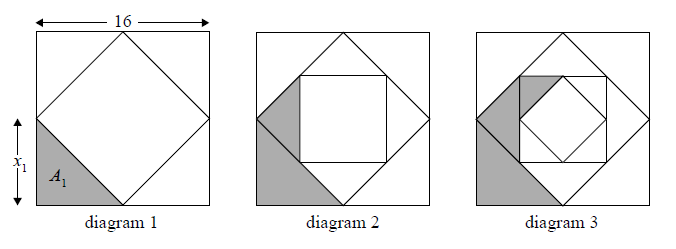
Let xnxn denote the length of one of the equal sides of each new triangle.
Let AnAn denote the area of each new triangle.
The following table gives the values of xnxn and AnAn, for 1⩽n⩽3. Copy and complete the table. (Do not write on this page.)
| n | 1 | 2 | 3 |
| xn | 8 | 4 | |
| An | 32 | 16 |
The process described above is repeated. Find A6.
Consider an initial square of side length k cm. The process described above is repeated indefinitely. The total area of the shaded regions is k cm2. Find the value of k.
Markscheme
valid method for finding side length (M1)
eg 82+82=c2, 45−45−90 side ratios, 8√2, 12s2=16, x2+x2=82
correct working for area (A1)
eg 12×4×4
| n | 1 | 2 | 3 |
| xn | 8 | √32 | 4 |
| An | 32 | 16 | 8 |
A1A1 N2N2
[4 marks]
METHOD 1
recognize geometric progression for An (R1)
eg un=u1rn−1
r=12 (A1)
correct working (A1)
eg 32(12)5; 4, 2, 1, 12, 14, …
A6=1 A1 N3
METHOD 2
attempt to find x6 (M1)
eg 8(1√2)5, 2√2, 2, √2, 1, …
x6=√2 (A1)
correct working (A1)
eg 12(√2)2
A6=1 A1 N3
[4 marks]
METHOD 1
recognize infinite geometric series (R1)
eg Sn=a1−r, |r|<1
area of first triangle in terms of k (A1)
eg 12(k2)2
attempt to substitute into sum of infinite geometric series (must have k) (M1)
eg 12(k2)21−12, k1−12
correct equation A1
eg 12(k2)21−12=k, k=k2812
correct working (A1)
eg k2=4k
valid attempt to solve their quadratic (M1)
eg k(k−4), k=4 or k=0
k=4 A1 N2
METHOD 2
recognizing that there are four sets of infinitely shaded regions with equal area R1
area of original square is k2 (A1)
so total shaded area is k24 (A1)
correct equation k24=k A1
k2=4k (A1)
valid attempt to solve their quadratic (M1)
eg k(k−4), k=4 or k=0
k=4 A1 N2
[7 marks]

