On this page, we will look at the properties of the reciprocal function and the rational function. You may be required to draw a sketch of these functions. In these cases, it is important to know how to find the vertical asymptote, the horizontal asymptote and the x and y intercepts.
On this page, you should learn about
the reciprocal function \(f(x)=\frac{1}{x}\) the rational function \(f(x)=\frac{ax+b}{cx+d}\) equations of vertical and horizontal asymptotes
Here is a quiz that practises the skills from this page
What is the equation of the vertical asymptote of the graph \(y=\frac{2x}{x-5}\)
The vertical asymptote occurs when x - 5 = 0
What is the equation of the horizontal asymptote of the graph \(y=\frac{2x}{x-5}\)
For the graph \(y=\frac{ax+b}{cx+d}\) , the horizontal asymptote is \(y=\frac{a}{c}\)
What is the y intercept of the function \(f(x)=\frac{2x-4}{5x+1}\)
The graph intersects the y axis when x = 0
\(\frac{0-4}{0+1}=-4\)
What is the x intercept of the function \(f(x)=\frac{2x-4}{5x+1}\)
The graph intersects the x axis when y = 0
\(\frac{2x-4}{5x+1}=0\)
2x - 4 = 0
x = 2
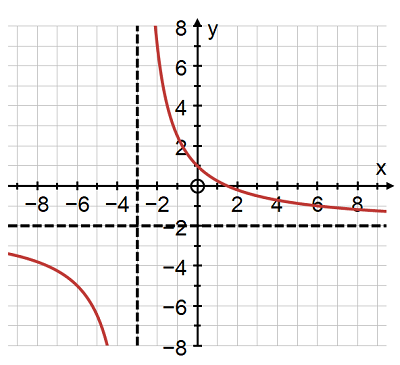
Which of the following graphs represents the function \(f(x)=-\frac{1}{x+1}\)
\(f(x)=-\frac{1}{x+1}\) has a vertical asymptote at x = -1
The y intercept is at \(y=-\frac{1}{0+1}=-1\)
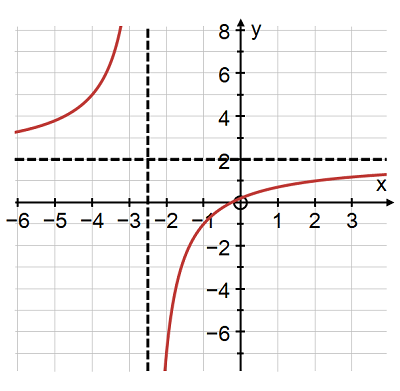
Which of the following graphs represents the function \(f(x)=\frac{3x + 2} {2x - 2}\)
\(f(x)=\frac{3x + 2} {2x - 2}\) has
a horizontal asymptote at y = \(\frac{3}{2}\)
a vertical asymptote where 2x - 2 = 0, that is x = 1
a y intercept at \(y=\frac{0+ 2} {0 - 2}=-1\)
The function \(f(x) =\frac{ax+1}{2x+b}\) is plotted
What are the values of a and b
The function
\(f(x) =\frac{ax+1}{2x+b}\) has
a vertical asymptote at \(x=-\frac{b}{2}\) a horizontal asymptote at \(y=\frac{a}{2}\) \(-\frac{b}{2}=2\) \(\frac{a}{2}=\frac{1}{2}\) b = - 4 a = 1
The function \(f(x) =\frac{ax+3}{bx+3}\) is plotted
What are the values of a and b
The function \(f(x) =\frac{ax+3}{bx+3}\) has
a vertical asymptote at \(x=-\frac{3}{b}\) a horizontal asymptote at \(y=\frac{a}{b}\) \(-\frac{3}{b}=-3\) \(\frac{a}{b}=-2\) b = 1 a = - 2
The function \(f(x) =\frac{4x+1}{ax+b}\) is plotted
What are the values of a and b
The function \(f(x) =\frac{4x+1}{ax+b}\) has
a horizontal asymptote at \(y=\frac{4}{a}\) a vertical asymptote at \(x=-\frac{b}{a}\) \(\frac{4}{a}=2\) \(-\frac{b}{2}=-\frac{5}{2}\) a = 2 b = 5
Which of the following correctly describes this function
This is a special rational function that has a hole at x = - 0.5
Hence the denominator of the rational function is 2x + 1
\(f(x)=\frac{4x+2}{2x+1},x\neq{-0.5}\)
\(f(x)=\frac{2(2x+1)}{2x+1},x\neq{-0.5}\)
\(f(x)=2,x\neq{-0.5}\)
Let f(x) = 2x + 1 and \(g(x)=\frac{x}{1-x} \ ,x\neq1\)
a) Show that \(f\circ g(x)=\frac{x+1}{1-x}\)
b) Let \(h(x)=\frac{x+1}{1-x}\) , for x < 1
c) Sketch the graph of h
d) Sketch the graph of \(h^{-1}\)
c) & d) In a sketch of a graph include
the equations of the asymptotes x intercept y intercept
Let \(f(x)=\frac{3x-2}{x-a},x\neq\ a\)
a) Find the inverse function \(f^{-1}(x)\) in terms of a
b) Find the value of a such that f
If f \(f(x)=f^{-1}(x)\)
The function f \(f(x)=\frac{6x+1}{2x-1},x\in\mathbb{R},x\neq\frac{1}{2}\)
a) Write f(x) \(A+\frac{B}{2x-1}\) where A and B are constants
b) Sketch the graph of f(x)
The horizontal asymptote is at x = A
MY PROGRESS
Self-assessment How much of Rational Functions SL have you understood?
My notes
Which of the following best describes your feedback?








 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn