| Date | None Specimen | Marks available | 8 | Reference code | SPNone.1.hl.TZ0.15 |
| Level | HL only | Paper | 1 | Time zone | TZ0 |
| Command term | Prove that | Question number | 15 | Adapted from | N/A |
Question
Prove the internal angle bisector theorem, namely that the internal bisector of an angle of a triangle divides the side opposite the angle into segments proportional to the sides adjacent to the angle.
The bisector of the exterior angle ˆA of the triangle ABC meets (BC) at P. The bisector of the interior angle ˆB meets [AC] at Q. Given that (PQ) meets [AB] at R, use Menelaus’ theorem to prove that (CR) bisects the angle AˆCB .
Markscheme
EITHER

let [AD] bisect A, draw a line through C parallel to (AD) meeting (AB) at E M1
then BˆAD=AˆEC and DˆAC=AˆCE A1
since BˆAD=DˆAC it follows that AˆEC=AˆCE A1
triangle AEC is therefore isosceles and AE=AC A1
since triangles BAD and BEC are similar
BDDC=ABAE=ABAC M1A1
OR
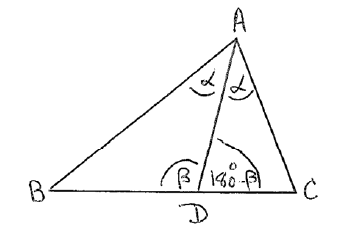
ABsinβ=BDsinα M1A1
ACsin(180−β)=DCsinα M1A1
sinβ=sin(180−β) R1
⇒ABBD=ACDC
⇒BDDC=ABAC A1
[6 marks]

using the angle bisector theorem, M1
AQQC=ABBC and BPPC=ABBC A1
using Menelaus’ theorem with (PR) as transversal to triangle ABC M1
BRAR×AQQC×PCBP=(−)1 A1
substituting the above results, M1
BRAR×ABBC×ACAB=(−)1 A1
giving
BRAR=BCAC A1
[CR] therefore bisects angle C by (the converse to) the angle bisector theorem R1AG
[8 marks]

