| Date | May 2010 | Marks available | 2 | Reference code | 10M.2.hl.TZ0.2 |
| Level | HL only | Paper | 2 | Time zone | TZ0 |
| Command term | Show that | Question number | 2 | Adapted from | N/A |
Question
The points D, E, F lie on the sides [BC], [CA], [AB] of the triangle ABC and [AD], [BE], [CF] intersect at the point G. You are given that CD =2BD and AG =2GD .
By considering (BE) as a transversal to the triangle ACD, show that
CEEA=32 .
Determine the ratios
(i) AFFB ;
(ii) BGGE .
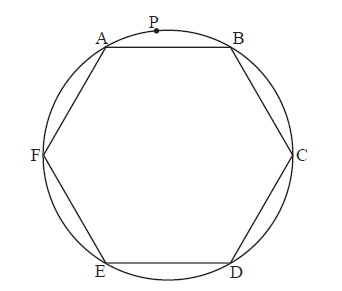
The diagram shows a hexagon ABCDEF inscribed in a circle. All the sides of the hexagon are equal in length. The point P lies on the minor arc AB of the circle. Using Ptolemy’s theorem, show thatPE+PD=PA+PB+PC+PF
Markscheme
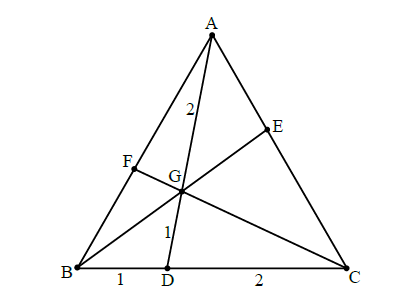
using Menelaus’ theorem in ΔACD ,
CEEA∙AGGD∙DBBC=−1 M1
CEEA∙21∙13=1 A1
CEEA=32 AG
[2 marks]
(i) using Ceva’s theorem in ΔABC ,
CEEA∙AFFB∙BDDC=1 M1
32∙AFFB∙12=1 A1
AFFB=43 A1
(ii) using Menelaus’ theorem in ΔABE , with traversal (FC), M1
AFFB∙BGGE∙ECCA=−1 A1
43∙BGGE∙35=1 A1
BGGE=54 A1
[7 marks]
using Ptolemy’s theorem in PAEC, M1
PA∙EC+AE∙PC=PE∙AC A1
since EC=AE=AC , M1
PE=PA+PC A1
similarly for PBDF, M1
PB∙DF+BD∙PF=PD∙BF (A1)
PD=PB+PF A1
adding these results,
PE+PD=PA+PB+PC+PF AG
[7 marks]
Examiners report
Part A was reasonably well done by many candidates. It would appear from the scripts that, in general, candidates find Menelaus’ Theorem more difficult to apply than Ceva’s Theorem, probably because the choice of transversal is not always obvious.
Part A was reasonably well done by many candidates. It would appear from the scripts that, in general, candidates find Menelaus’ Theorem more difficult to apply than Ceva’s Theorem, probably because the choice of transversal is not always obvious.
Few fully correct answers were seen to part B with most candidates unable to identify which cyclic quadrilaterals should be used.

