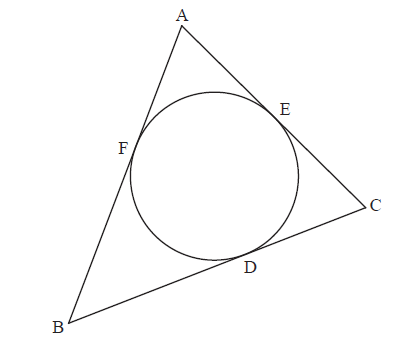
The diagram shows triangle ABC together with its inscribed circle. Show that [AD], [BE] and [CF] are concurrent.

PQRS is a parallelogram and T is a point inside the parallelogram such that the sum of ${\rm{P}}\hat {\rm{T}}{\rm{Q}}$ and ${\rm{R}}\hat {\rm{T}}{\rm{S}}$ is ${180^ \circ }$ . Show that ${\rm{TP}} \times {\rm{TR}} + {\rm{ST}} \times {\rm{TQ}} = {\rm{PQ}} \times {\rm{QR}}$ .

Since the lengths of the two tangents from a point to a circle are equal     (M1)

${\rm{AF = AE, BF = BD, CD = CE}}$     A1

Consider

$\frac{{{\rm{AF}}}}{{{\rm{FB}}}} \times \frac{{{\rm{BD}}}}{{{\rm{DC}}}} \times \frac{{{\rm{CE}}}}{{{\rm{EA}}}} = 1$ (signed lengths are not relevant here)     M2A2

It follows by the converse to Ceva’s Theorem that [AD], [BE], [CF] are concurrent.     R2

[8 marks]

Draw the $\Delta {\rm{PQU}}$ congruent to $\Delta {\rm{SRT}}$ (or translate $\Delta {\rm{SRT}}$ to form $\Delta {\rm{PQU}}$ ).     R2

Since ${\rm{P}}\hat {\rm{U}}{\rm{Q}} = {\rm{S}}\hat {\rm{T}}{\rm{R}}$ , and ${\rm{S}}\hat {\rm{T}}{\rm{R + P}}\hat {\rm{T}}{\rm{Q}} = {180^ \circ }$ it follows that     R2  

${\rm{P}}\hat {\rm{U}}{\rm{Q + P}}\hat {\rm{T}}{\rm{Q}} = {180^ \circ }$     R1

The quadrilateral PUQT is therefore cyclic     R2

Using Ptolemy’s Theorem,     M2

${\rm{UQ}} \times {\rm{PT}} + {\rm{PU}} \times {\rm{QT}} = {\rm{PQ}} \times {\rm{UT}}$     A2

Since ${\rm{UQ = TR}}$ , ${\rm{PU = ST}}$ and ${\rm{UT = QR}}$     R2

Then ${\rm{TP}} \times {\rm{TR}} + {\rm{ST}} \times {\rm{TQ}} = {\rm{PQ}} \times {\rm{QR}}$     AG

[13 marks]