| Date | November 2015 | Marks available | 5 | Reference code | 15N.1.sl.TZ0.8 |
| Level | SL only | Paper | 1 | Time zone | TZ0 |
| Command term | Find | Question number | 8 | Adapted from | N/A |
Question
The following diagram shows part of the graph of a quadratic function f.
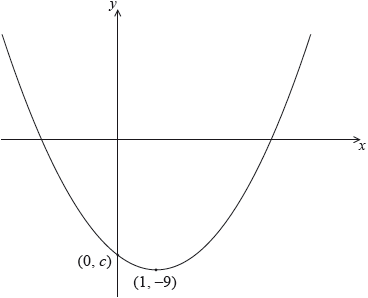
The vertex is at (1, −9), and the graph crosses the y-axis at the point (0, c).
The function can be written in the form f(x)=(x−h)2+k.
Write down the value of h and of k.
Find the value of c.
Let g(x)=−(x−3)2+1. The graph of g is obtained by a reflection of the graph of f in the x-axis, followed by a translation of (pq).
Find the value of p and of q.
Find the x-coordinates of the points of intersection of the graphs of f and g.
Markscheme
h=1, k=−9(accept (x−1)2−9) A1A1 N2
[2 marks]
METHOD 1
attempt to substitute x=0 into their quadratic function (M1)
egf(0), (0−1)2−9
c=−8 A1 N2
METHOD 2
attempt to expand their quadratic function (M1)
egx2−2x+1−9, x2−2x−8
c=−8 A1 N2
[2 marks]
evidence of correct reflection A1
eg−((x−1)2−9), vertex at (1, 9), y-intercept at (0, 8)
valid attempt to find horizontal shift (M1)
eg1+p=3, 1→3
p=2 A1 N2
valid attempt to find vertical shift (M1)
eg9+q=1, 9→1, −9+q=1
q=−8 A1 N2
Notes: An error in finding the reflection may still allow the correct values of p and q to be found, as the error may not affect subsequent working. In this case, award A0 for the reflection, M1A1 for p=2, and M1A1 for q=−8.
If no working shown, award N0 for q=10.
[5 marks]
valid approach (check FT from (a)) M1
egf(x)=g(x), (x−1)2−9=−(x−3)2+1
correct expansion of both binomials (A1)
egx2−2x+1, x2−6x+9
correct working (A1)
egx2−2x−8=−x2+6x−8
correct equation (A1)
eg2x2−8x=0, 2x2=8x
correct working (A1)
eg2x(x−4)=0
x=0, x=4 A1A1 N3
[7 marks]
Total [16 marks]

