| Date | May 2012 | Marks available | 2 | Reference code | 12M.1.sl.TZ2.5 |
| Level | SL only | Paper | 1 | Time zone | TZ2 |
| Command term | Sketch | Question number | 5 | Adapted from | N/A |
Question
The diagram below shows the graph of a function f(x) , for −2≤x≤3 .
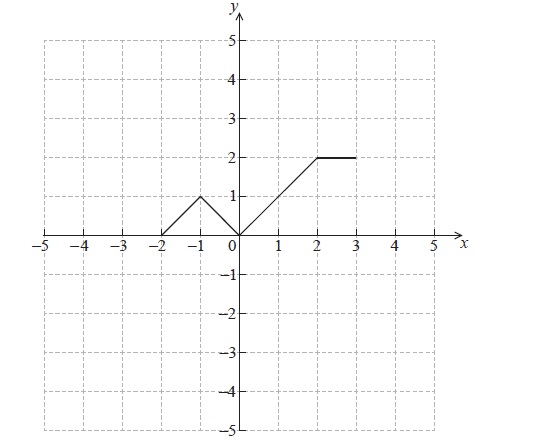
Sketch the graph of f(−x) on the grid below.

The graph of f is transformed to obtain the graph of g . The graph of g is shown below.
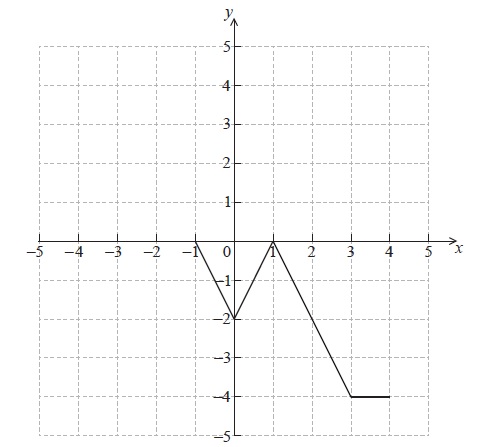
The function g can be written in the form g(x)=af(x+b) . Write down the value of a and of b .
Markscheme
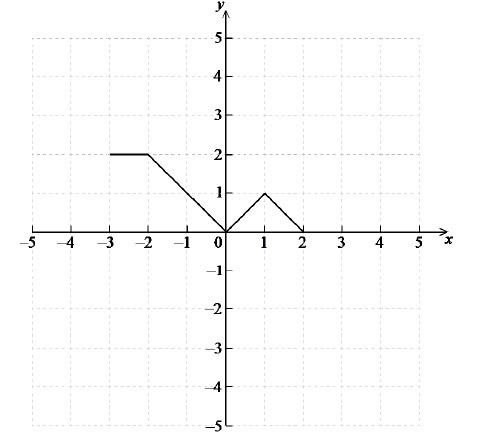 A2 N2
A2 N2
[2 marks]
a=−2,b=−1 A2A2 N4
Note: Award A1 for a=2 , A1 for b=1 .
[4 marks]
Examiners report
In part (a) of this question, a large number of candidates correctly sketched the graph of f(−x) , as asked. A fairly common error, however, was to graph −f(x) .
In part (b), many candidates seemed to recognize that the value of a was related to a vertical stretch, though some omitted the negative required for the vertical reflection. Similarly, some candidates gave a positive value for b.

