find the value of $\cos x;$

find the value of $\cos 2x.$

valid approach     (M1)

eg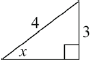$\;\;\;$, ${\sin ^2}x + {\cos ^2}x = 1$

correct working     (A1)

eg$\;\;\;{4^2} - {3^2},{\text{ }}{\cos ^2}x = 1 - {\left( {\frac{3}{4}} \right)^2}$

correct calculation     (A1)

eg$\;\;\;\frac{{\sqrt 7 }}{4},{\text{ }}{\cos ^2}x = \frac{7}{{16}}$

$\cos x =  - \frac{{\sqrt 7 }}{4}$     A1     N3

[4 marks]

correct substitution (accept missing minus with cos)     (A1)

eg$\;\;\;1 - 2{\left( {\frac{3}{4}} \right)^2},{\text{ }}2{\left( { - \frac{{\sqrt 7 }}{4}} \right)^2} - 1,{\text{ }}{\left( {\frac{{\sqrt 7 }}{4}} \right)^2} - {\left( {\frac{3}{4}} \right)^2}$

correct working     A1

eg$\;\;\;2\left( {\frac{7}{{16}}} \right) - 1,{\text{ }}1 - \frac{{18}}{{16}},{\text{ }}\frac{7}{{16}} - \frac{9}{{16}}$

$\cos 2x =  - \frac{2}{{16}}\;\;\;\left( { =  - \frac{1}{8}} \right)$     A1     N2

[3 marks]

Total [7 marks]

Many candidates were able to find the cosine ratio of $\frac{{\sqrt 7 }}{4}$ but did not take into account the information about the obtuse angle and seldom selected the negative answer. Finding $\cos 2x$ proved easier; the most common error seen was $\cos 2x = 2\cos x$.

Many candidates were able to find the cosine ratio of $\frac{{\sqrt 7 }}{4}$ but did not take into account the information about the obtuse angle and seldom selected the negative answer. Finding $\cos 2x$ proved easier; the most common error seen was $\cos 2x = 2\cos x$.