Given that \(\cos A = \frac{1}{3}\) and \(0 \le A \le \frac{\pi }{2}\) , find \(\cos 2A\) .

Given that \(\sin B = \frac{2}{3}\)  and \(\frac{\pi }{2} \le B \le \pi \) , find \(\cos B\) .

evidence of choosing the formula \(\cos 2A = 2{\cos ^2}A - 1\)     (M1)

Note: If they choose another correct formula, do not award the M1 unless there is evidence of finding \({\sin ^2}A = 1 - \frac{1}{9}\)

correct substitution     A1

 e.g.\(\cos 2A = {\left( {\frac{1}{3}} \right)^2} - \frac{8}{9}\) , \(\cos 2A = 2 \times {\left( {\frac{1}{3}} \right)^2} - 1\)

 \(\cos 2A = - \frac{7}{9}\)     A1     N2

[3 marks]

METHOD 1

evidence of using \({\sin ^2}B + {\cos ^2}B = 1\)     (M1)

e.g. \({\left( {\frac{2}{3}} \right)^2} + {\cos ^2}B = 1\) , \(\sqrt {\frac{5}{9}} \) (seen anywhere),

\(\cos B = \pm \sqrt {\frac{5}{9}} \) \(\left( { = \pm \frac{{\sqrt 5 }}{3}} \right)\)     (A1)

 \(\cos B = - \sqrt {\frac{5}{9}} \) \(\left( { = - \frac{{\sqrt 5 }}{3}} \right)\)     A1     N2

METHOD 2

diagram     M1

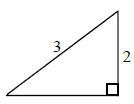
for finding third side equals \(\sqrt 5 \)     (A1)

\(\cos B = - \frac{{\sqrt 5 }}{3}\)     A1     N2

[3 marks]

This question was very poorly done, and knowledge of basic trigonometric identities and values of trigonometric functions of obtuse angles seemed distinctly lacking. Candidates who recognized the need of an identity for finding \(\cos 2A\) given \(\cos A\) seldom chose the most appropriate of the three and even when they did often used it incorrectly with expressions such as \(2{\cos ^2}\frac{1}{9} - 1\) .

This question was very poorly done, and knowledge of basic trigonometric identities and values of trigonometric functions of obtuse angles seemed distinctly lacking. Candidates who recognized the need of an identity for finding \(\cos 2A\) given \(\cos A\) seldom chose the most appropriate of the three and even when they did often used it incorrectly with expressions such as \(2{\cos ^2}\frac{1}{9} - 1\) .