Find \(\cos \theta \) .

Find \(\tan 2\theta \) .

METHOD 1

evidence of choosing \({\sin ^2}\theta  + {\cos ^2}\theta  = 1\)     (M1)

correct working     (A1)

e.g. \({\cos ^2}\theta  = \frac{9}{{13}}\) , \(\cos \theta  =  \pm \frac{3}{{\sqrt {13} }}\) , \(\cos \theta  = \sqrt {\frac{9}{{13}}} \)

\(\cos \theta  = - \frac{3}{{\sqrt {13} }}\)    A1     N2

Note: If no working shown, award N1 for \(\frac{3}{{\sqrt {13} }}\) .

METHOD 2

approach involving Pythagoras’ theorem     (M1)

e.g. \({2^2} + {x^2} = 13\) ,

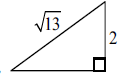
finding third side equals 3     (A1)

\(\cos \theta  = - \frac{3}{{\sqrt {13} }}\)     A1     N2

Note: If no working shown, award N1 for \(\frac{3}{{\sqrt {13} }}\) .

[3 marks]

correct substitution into \(\sin 2\theta \) (seen anywhere)     (A1)

e.g. \(2\left( {\frac{2}{{\sqrt {13} }}} \right)\left( { - \frac{3}{{\sqrt {13} }}} \right)\)

correct substitution into \(\cos 2\theta \) (seen anywhere)     (A1)

e.g. \({\left( { - \frac{3}{{\sqrt {13} }}} \right)^2} - {\left( {\frac{2}{{\sqrt {13} }}} \right)^2}\) , \(2{\left( { - \frac{3}{{\sqrt {13} }}} \right)^2} - 1\) , \(1 - 2{\left( {\frac{2}{{\sqrt {13} }}} \right)^2}\)

valid attempt to find \(\tan 2\theta \)     (M1)

e.g. \(\frac{{2\left( {\frac{2}{{\sqrt {13} }}} \right)\left( { - \frac{3}{{\sqrt {13} }}} \right)}}{{{{\left( { - \frac{3}{{\sqrt {13} }}} \right)}^2} - {{\left( {\frac{2}{{\sqrt {13} }}} \right)}^2}}}\) , \(\frac{{2\left( { - \frac{2}{3}} \right)}}{{1 - {{\left( { - \frac{2}{3}} \right)}^2}}}\)

correct working     A1

e.g. \(\frac{{\frac{{(2)(2)( - 3)}}{{13}}}}{{\frac{9}{{13}} - \frac{4}{{13}}}}\) , \(\frac{{ - \frac{{12}}{{{{\left( {\sqrt {13} } \right)}^2}}}}}{{\frac{{18}}{{13}} - 1}}\) , \(\frac{{ - \frac{{12}}{{13}}}}{{\frac{5}{{13}}}}\)

\(\tan 2\theta  = - \frac{{12}}{5}\)     A1     N4

Note: If students find answers for \(\cos \theta \) which are not in the range \([ - 1{\text{, }}1]\), award full FT in (b) for correct FT working shown.

[5 marks]

While the majority of candidates knew to use the Pythagorean identity in part (a), very few remembered that the cosine of an angle in the second quadrant will have a negative value.

In part (b), many candidates incorrectly tried to calculate \(\tan 2\theta \) as \(2 \times \tan \theta \) , rather than using the double-angle identities.