Find ${h^{ - 1}}(x)$ .

(i)     Sketch the graph of h for $- 4 \le x \le 4$ and $- 5 \le y \le 8$ , including any asymptotes.

(ii)    Write down the equations of the asymptotes.

(iii)   Write down the x-intercept of the graph of h .

Let R be the region in the first quadrant enclosed by the graph of h , the x-axis and the line $x = 3$.

(i)     Find the area of R.

(ii)    Write down an expression for the volume obtained when R is revolved through ${360^ \circ }$ about the x-axis.

$y = \frac{{2x - 1}}{{x + 1}}$

interchanging x and y (seen anywhere)     M1

e.g. $x = \frac{{2y - 1}}{{y + 1}}$

correct working     A1

e.g. $xy + x = 2y - 1$

collecting terms     A1

e.g. $x + 1 = 2y - xy$ , $x + 1 = y(2 - x)$

${h^{ - 1}}(x) = \frac{{x + 1}}{{2 - x}}$     A1     N2

[4 marks]

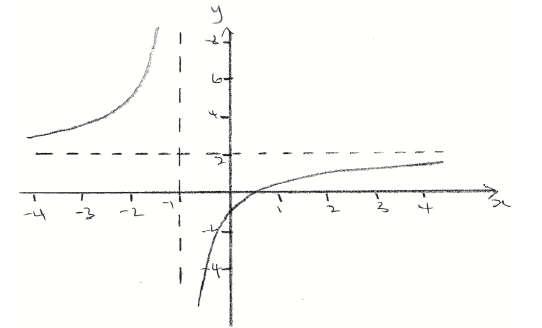     A1A1A1A1     N4

Note: Award A1 for approximately correct intercepts, A1 for correct shape, A1 for asymptotes, A1 for approximately correct domain and range.

(ii) $x = - 1$ , $y = 2$     A1A1     N2

(iii) $\frac{1}{2}$     A1     N1

[7 marks]

(i) ${\text{area}} = 2.06$     A2     N2

(ii) attempt to substitute into volume formula (do not accept $\pi \int_a^b {{y^2}{\rm{d}}x}$ )     M1

volume $= \pi {\int_{\frac{1}{2}}^3 {\left( {\frac{{2x - 1}}{{x + 1}}} \right)} ^2}{\rm{d}}x$     A2     N3

[5 marks]